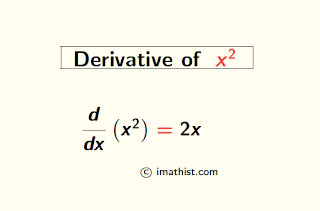The derivative of x2 (x square) by first principle is 2x. The function x2 denotes the square of x. In this post, we will find the derivative of x square.
The derivative of x square is denoted by d/dx (x2), and its formula is given below:
$\dfrac{d}{dx}$ (x2) = 2x.
Derivative of x2 by First Principle
Recall the first principle of derivatives: The derivative of a function f(x) by first principle is given as follows.
$\dfrac{d}{dx}(f(x))$ = limh→0 $\dfrac{f(x+h)-f(x)}{h}$
Let f(x)=x2
So the derivative of x square by the first principle is equal to
$\dfrac{d}{dx}(x^2)$ $=\lim\limits_{h \to 0} \dfrac{(x+h)^2-x^2}{h}$
= limh→0 $\dfrac{x^2+2xh+h^2-x^2}{h}$ using the algebraic identity (a+b)2 = a2+2ab+b2.
= limh→0 $\dfrac{2xh+h^2}{h}$
= limh→0 $\dfrac{h(2x+h)}{h}$
= limh→0 $[2x+h]$
= 2x+0
= 2x.
Hence, the derivative of x2 by first principle is 2x.
More Derivatives: Derivative of x5 by First Principle
Derivative of esinx by First Principle
Derivative of x6 by First Principle
Derivative of x2 by Power Rule
Let us use the power rule of derivatives to find the derivative of x square. The power rule says that the derivative of xn is given by $\dfrac{d}{dx}(x^n)$=nxn-1.
Putting n=2 in the above rule, we will get the derivative of x2. Thus, we have that
$\dfrac{d}{dx}(x^2)$ =2x2-1 = 2x1 =2x.
Hence, the derivative of x2 (x square) by the power rule of derivatives is 2x.
More Reading: Derivative of xn
Derivative of 2x | Derivative of x10
Question-Answer
Question 1: Find the derivative of $(\ln x)^2$
Solution:
Let z=ln x.
So we have $\dfrac{dz}{dx}=\dfrac{1}{x}$.
Then by the chain rule of derivatives, the derivative of \lnx square is equal to
$\dfrac{d}{dx}(z^2)$ $=\dfrac{d}{dz}(z^2) \cdot \dfrac{dz}{dx}$
$=2z \cdot \dfrac{1}{x}$
$=\dfrac{2}{x} \ln x$ as z=ln x.
Thus, the derivative of (ln x)2 is equal to (2/x) ln x.
Also Read:
Derivative of root x + 1 by root x
FAQs
Q1: What is the derivative x2?
Answer: The derivative of x^2 is equal to 2x.