[Solved] What is the Derivative of pi (π)?

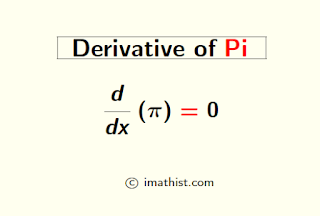
The derivative of pi is zero, that is, d/dx(π)=0. Note that pi is denoted by π. In this post, we will learn how to find the derivative of π.

Derivative of pi Formula

The formula for the derivative of $\pi$ is $0$. This formula can be written as follows:

$\dfrac{d}{dx}(\pi)=0$ or $(\pi)’=0$.

Here, the prime $’$ denotes the first-order derivative.

What is the Derivative of pi?

Answer: The derivative of pi is $0$.

Explanation:

Value of pi: The number $\pi$ is irrational, and its value is approximately equal to 3.1415926536 (up to $10$ decimal places).

We know that the value of $\pi$ is given by the area of a circle of unit radius (that is, of radius 1). Note that the area of a circle of radius $r$ unit is equal to $\pi r^2$ $\text{unit}^2$.

As the area of a unit circle is fixed, we conclude that $\pi$ is a fixed number. Thus, $\pi$ is a constant and so it does not change with respect to any variable.

$\therefore \dfrac{d}{dx}(\pi)=0$ by the rule Derivative of a constant is 0.

Thus, the derivative of $\pi$ is equal to $0$.

Also Read:

Derivative of e^2

Derivative of e^3

Derivative of e^3x

Derivative of log3x

Derivative of pi by First Principle

Let $f(x)=\pi$. See that $\pi$ is independent of $x$, so we have $f(x+h)=\pi$ for any values of $x$ and $h$. Now, by the first principle, the derivative of $f(x)$ is equal to

$\dfrac{d}{dx}(f(x))$ $=\lim\limits_{h \to 0} \dfrac{f(x+h)-f(x)}{h}$

Put $f(x)=\pi$.

So $\dfrac{d}{dx}(\pi)$ $=\lim\limits_{h \to 0} \dfrac{\pi-\pi}{h}$

$=\lim\limits_{h \to 0} \dfrac{0}{h}$

$=\lim\limits_{h \to 0} 0$ $=0$.

Thus, the derivative of  $\pi$ using the first principle, that is, by the limit definition is equal to $0$.

Also Read:

Derivative of sin 4x

Derivative of tan 3x

FAQs