The integration of cosec x is ln|cosec x-cot x|. Here ln stands for the natural logarithm, that is, ln x = logex. In this post, we will learn how to integrate cosec x.
Integration of Cosecx Formula
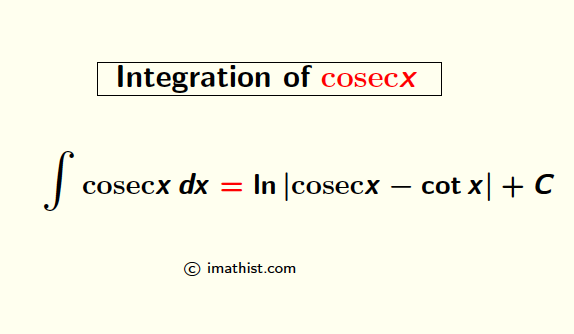
Cosecx integration formula: The integration formula of cosec x is given below.
∫cosecx dx = ln|cosecx-cotx|+C
where C is an integral constant.
Integration of Cosecx Proof
We will prove that ∫cosecx dx = ln|cosecx-cotx|+C. Note that
∫cosecx dx = ∫cosecx × 1 dx
= ∫cosecx $\times \dfrac{\text{cosec} x -\cot x}{\text{cosec} x – \cot x}$ dx
= ∫ $\dfrac{\text{cosec}^2 x – \text{cosec} x\cot x}{\text{cosec} x – \cot x}$ dx …(I)
Put cosecx – cotx = t.
Differentiating both sides, we have
(-cosec x cot x+cosec2x)dx = dt.
Thus, we have from (I) that
∫cosecx dx = ∫ $\dfrac{dt}{t}$
= ln|t|+C where C is an integral constant, and ln stands for the natural logarithm which is loge.
= ln|cosecx – cotx|+C as t=cosecx – cotx.
So the integration of cosecx is ln|cosecx – cotx|+C where C is an integral constant. This is derived by the substitution method of integration.
Video Solution on Integration of cosecx:
ALSO READ:
FAQs
Q1: What is the integration of cosecx?
Answer: The integration of cosecx is equal to ln|cosecx – cotx|+C.