The simplification or the formula of 1+cot2x is given as follows:
1+cot2x = cosec2x.
In this post, we will learn how to establish the formula of 1+cot2x.
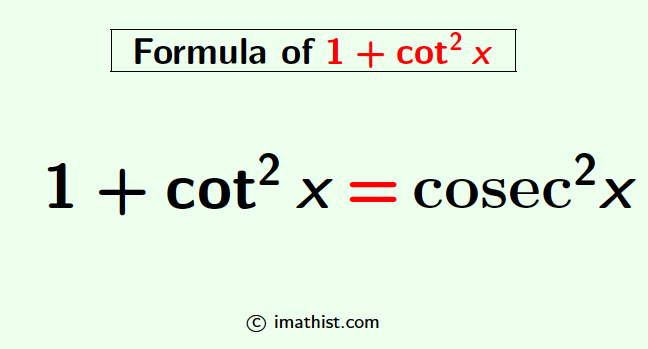
Proof of 1+cot2x Formula
Question: Prove the formula 1+cot2x = cosec2x.
Solution:
L.H.S. = 1+cot2x
= $1+(\dfrac{\cos x}{\sin x})^2$ as cotx=cosx/sinx.
= $1+\dfrac{\cos^2 x}{\sin^2 x}$
= $\dfrac{\sin^2 x + \cos^2 x}{\sin^2 x}$
= $\dfrac{1}{\sin^2 x}$ as we know that sin2x+cos2x=1.
= $(\dfrac{1}{\sin x})^2$
= cosec2x [∵ cosecx = 1/sinx]
= R.H.S.
So we have proven the formula of 1+cot2x in terms of cosec which is given by 1+cot2x = cosec2x.
Question-Answer
Question: Find the value of 1+cot245.
Answer:
In the above formula of 1+cot2x = cosec2x, we put x=45 degrees. So we get that
1+cot245 = cosec245 = (√2)2 = 2.
So the value of 1+cot245 is equal to 2.
ALSO READ:
FAQs
Q1: What is the formula of 1+cot2x?
Answer: The formula of 1+cot2x is equal to 1+cot2x=cosec2x.
Q2: What is the formula of 1+cot2θ?
Answer: The formula of 1+cot2θ is equal to 1+cot2θ=cosec2θ.