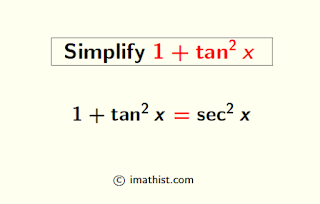Simplify the expression 1+tan^2 x | 1+tan^2x Formula

The simplification of 1+tan² x is equal to sec² x. In this post, we will find the formula of 1+tan²x. This will be proved using trigonometric identities.

1+tan²x Formula

To simplify the expression 1+tan² x, we will follow the below steps:

Step 1: At first, we put $\tan x=\dfrac{\sin x}{\cos x}$. By doing so, we obtain that

$1+\tan^2 x$ $=1+(\dfrac{\sin x}{\cos x})^2$

$=1+\dfrac{\sin^2 x}{\cos^2 x}$

Step 2: Simplifying the above, we get that

$1+\tan^2 x=1+\dfrac{\sin^2 x}{\cos^2 x}$

$=\dfrac{\sin^2 x+\cos^2 x}{\cos^2 x}$

$=\dfrac{1}{\cos^2 x}$ as we know that $\sin^2 x+\cos^2 x=1$

$=(\dfrac{1}{\cos x})^2$ $=(\sec x)^2$ as the reciprocal of cosx is secx.

$=\sec^2 x$

Conclusion: Thus, the simplification of $1+\tan^2 x$ is equal to $\sec^2 x$.

Note that we also have that $1+\tan^2 \theta=\sec^2 \theta$ obtained in a similar way as above.

Also Read:

sinx=0, cosx=0, tanx=0 General Solution

Simplify cos(x-pi)

Values of sin 15, cos 15, tan 15

Values of sin 75, cos 75, tan 75

Question-Answer on 1+tan²x Formula

Question 1: Find the value of 1+tan² $45^\circ$

Answer:

From the above, we get that the value of 1+tan² x is equal to sec² x. In this formula, we put $x=45^\circ$. Thus, we have

$1+\tan^2 45^\circ$ $=\sec^2 45^\circ$

$=\dfrac{1}{\cos^2 45^\circ}$

$=\dfrac{1}{(\dfrac{1}{\sqrt{2}})^2}$ as we know that $\cos 45^\circ =\dfrac{1}{\sqrt{2}}$.

$=\dfrac{1}{1/2}=2$

Thus, the value of $1+\tan^2 45^\circ$ is equal to $2$.

FAQs