Derivative of root cosx by Chain Rule

The derivative of root cosx is equal to -sinx/(2√cosx).  In this post, we will learn how to differentiate the square root of cos x by the chain rule of derivatives.

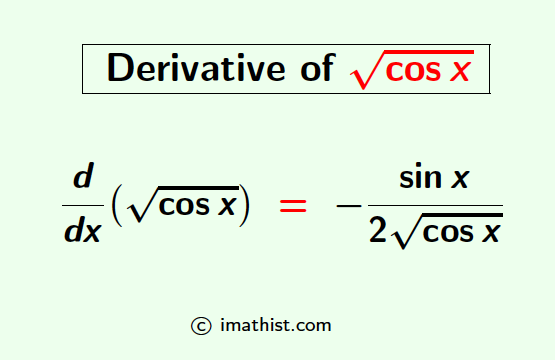
Derivative of Square Root of cos x by Chain Rule

Question: Find the derivative of  $\sqrt{\cos x}$ by the chain rule.

Solution:

To find the derivative of root cosx by the chain rule, we will follow the below steps provided here.

Step 1:

Let u=cosx

Differentiating both sides with respect to x, we have

$\dfrac{du}{dx} = -\sin x$

Step 2:

Now, applying the chain rule of derivatives, we get that

$\dfrac{d}{dx}(\sqrt {\cos x})$ = $\dfrac{d}{dx}(\sqrt u)$

= $\dfrac{d}{du}(\sqrt{u}) \times \dfrac{du}{dx}$

= $\dfrac{d}{du}(u^{1/2}) \times (-\sin x)$ from step1

= ½ u^{½ -1} × (-sin x) by the power rule of derivatives $\frac{d}{dx}$(xⁿ)=nx^n-1.

Step 3:

Lastly, we put u=cosx and simplify. So we have

$\dfrac{d}{dx}(\sqrt {\cos x})$ = $\dfrac{-\sin x}{2 \sqrt{u}}$, this is because u^-1/2=1/√u.

= $-\dfrac{\sin x}{2 \sqrt{\cos x}}$

So the derivative of the square root of cos x is equal to $\dfrac{-\sin x}{2 \sqrt{\cos x}}$ which is obtained by the chain rule of derivatives.

Also Read:

Derivative of $\sqrt{\sin x}$ by First Principle

Derivative of $\sqrt{\sin x}$ by Chain Rule

Derivative of $\sqrt{\cos x}$

Derivative of $\sqrt{e^x}$

FAQs