What is the Integration of cot^2x | Integral of cot^2 x

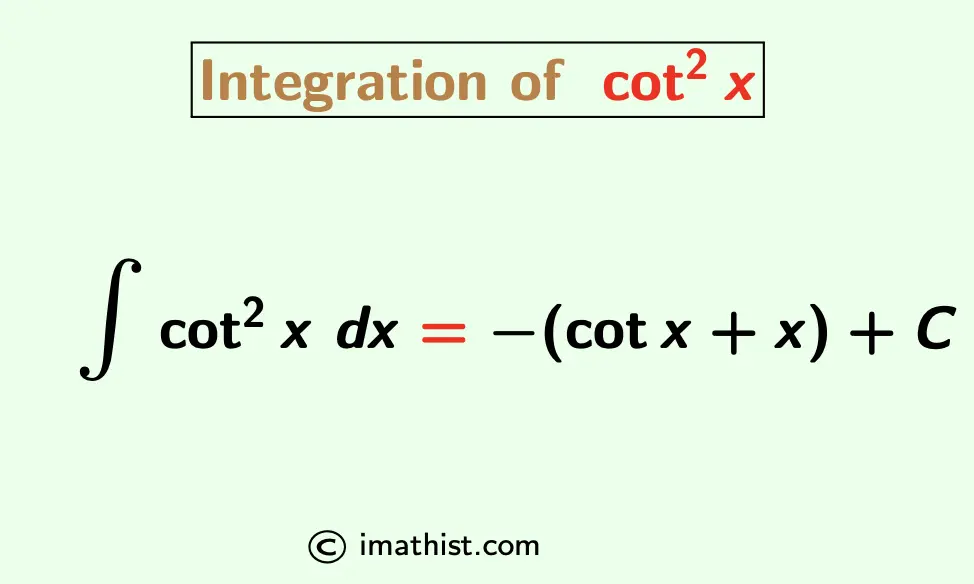
The integration of cot^2x is equal to -(cot x +x)+C. In this post, we will learn how to find the integral of cot^2x (cot square x). We will use the following two formulas:

1. ∫cosec²x dx = -cot x+C
2. ∫dx = x+C.

Find Integration of cot²x

Question: Find the integration of cot2 x? That is, find ∫cot2 x dx.

Answer: The integration of cot²x is given by the formula ∫cot²x dx = -(cotx +x)+C.

Explanation:

Recall the following trigonometric formula:

cosec² x =1+cot² x

∴ cot² x = cosec² x -1

Therefore, integrating both sides we obtain that

∫cot²x dx = ∫(cosec² x-1) dx +C

⇒ ∫cot²x dx = ∫cosec² x dx – ∫dx +C

⇒ ∫cot²x dx = -cotx – x+C by the above two formulas.

So the integration of cot^2x (cot square x) is equal to -(cotx +x)+C where C denotes an arbitrary integral constant.

ALSO READ:

Integration of tan2x	The integration of tan2x is ∫tan2x dx = tanx -x+C.
Integration of sin2x	The integration of sin2x is ∫sin2x dx = x/2-(sin2x)/4+C
Integration of cos2x	The integration of cos2x is ∫cos2x dx = x/2+(sin2x)/4+C

Definite Integral of cot²x

Question: Find the definite integral of cot²x from π/4 to π/2, that is,

find $\int_{\frac{\pi}{4}}^{\frac{\pi}{2}} \cot^2 x dx$

Solution:

From the above, we have ∫cot²x dx = -cot x – x +C. Therefore, we get that

$\int_{\frac{\pi}{4}}^{\frac{\pi}{2}} \cot^2 x dx$

= [-cot x -x+C]$_{\frac{\pi}{4}}^{\frac{\pi}{2}}$

= [-cot(π/2) –  π/2 +C] – [-cot(π/4) –  π/4 +C]

= -0 –  π/2 +C +1 +π/4 -C as the value of cot(π/2) is 0 and the value of cot(π/4) is 1.

= 1- π/4

= (4-π)/4.

Thus, the definite integral of cot²x from π/4 to π/2 is equal to (4-π)/4.

Have You Read These Integrals?

Integration of sin3x	Integration of e3x
Integration of log(sin x)	Integration of 2x

FAQs