Cos^3x Formula, Proof | Cos Cube x Formula

The cos^3x formula is given by cos³x = (cos3x + 3cosx)/4. In this post, we learn how to prove the cos cube x formula.

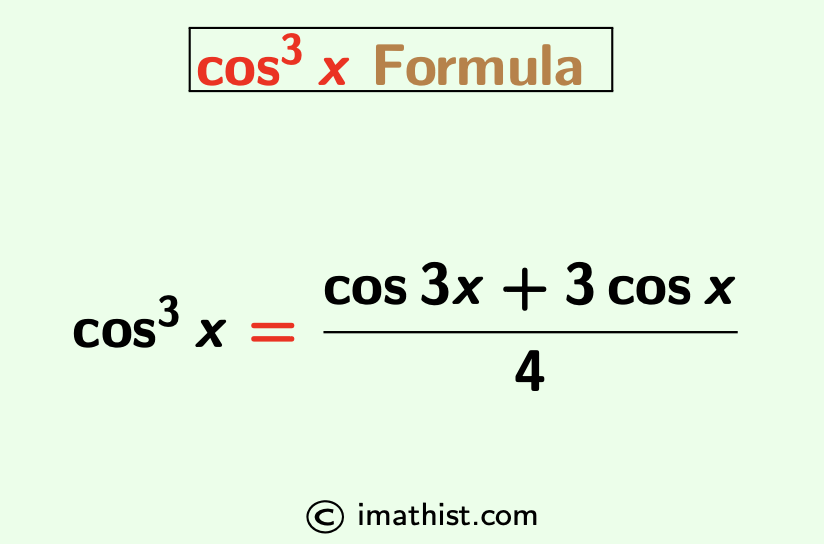
What is cos cube x formula

Answer: cos3x=(cos3x + 3cosx)/4 is the formula of cos cube x.

Proof:

We will follow the below steps in order to prove cos cube x formula.

Step 1: First, we use cos3x formula and the formula is given below:

cos3x = 4cos³x – 3cos x.

Step 2: Now we add 3cosx to both sides of the above equation. By doing so, we obtain that

cos3x + 3cox= 4cos³x – 3cos x +3cosx.

⇒ cos3x + 3cox = 4cos³x …(I)

Step 3: Dividing both sides of (I) by 4, we have that

(cos3x + 3cosx)/4 = cos³x.

Therefore, the cos³x formula is obtained as follows: cos³x = (cos3x + 3cosx)/4.

Remark:

Replacing x in the above formula by θ, the formula of cos³θ (cos cube theta) is given by cos³θ = (cos3θ + 3cosθ)/4.

Have You Read These? 

Sin3x Formula	Cos3x Formula
sin3x Formula	sinx siny Identity
tan(x-y) Formula	cot(x+y) Formula
Simplify sin(cos-1x)	cos2x-sin2x Formula

FAQs