Find the Derivative of 3/x

The derivative of 3/x is equal to -3/x². The derivative formula of 3/x (3 divided by x) is given by as follows:

$\dfrac{d}{dx}(\dfrac{3}{x})= – \dfrac{3}{x^2}$.

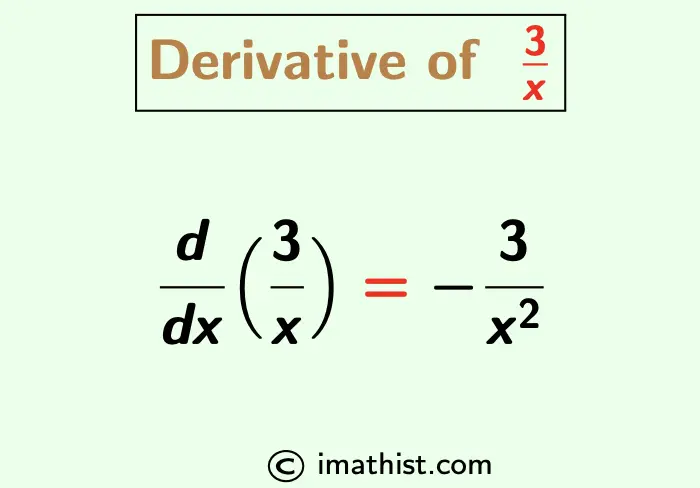
Derivative of 3/x

Question: What is the Derivative of 3/x?

Answer: As 3/x can be expressed as 3x^-1, applying the power rule, the derivative of 3/x is equal to -3/x².

Explanation:

$\dfrac{d}{dx}(\dfrac{3}{x})$ = $\dfrac{d}{dx}(3x^{-1})$

= $3 \dfrac{d}{dx}(x^{-1})$

= 3 × -x^-1-1 by the power rule of derivatives

= -3x^-2

= $-\dfrac{3}{x^2}$.

So the derivative of 3/x is equal to -3/x², and this is proved by the power rule of derivatives.

Related Derivatives:

Derivative of 1/x	Derivative of 2/x
Derivative of 1/x2	Derivative of 1/2x
Derivative of 2x	Derivative of 10x

FAQs