Find the Derivative of 1/x^n (1 by x power n)

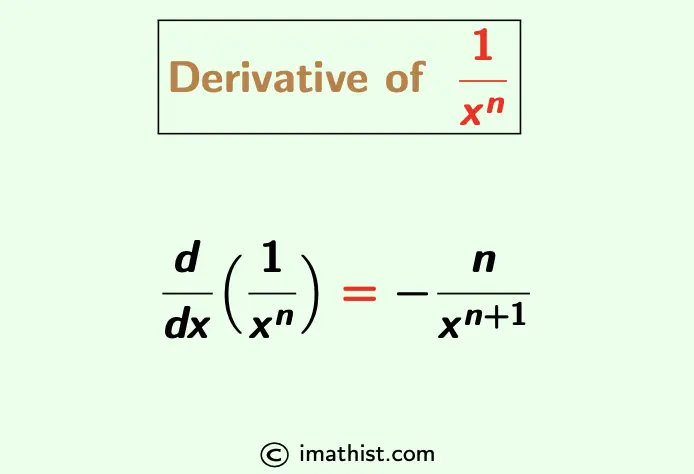
The derivative of 1/xⁿ (1 by x power n) is equal to -n/xⁿ⁺¹. Here we learn how to find the derivative of 1 divided by x to the n.

The derivative formula of 1/x^n is given by

$\dfrac{d}{dx} \big( \dfrac{1}{x^n}\big) = -\dfrac{n}{x^{n+1}}$.

Derivative of 1/xⁿ

Answer: The derivative of 1/xⁿ is equal to -n/xⁿ⁺¹.

Explanation:

By the rule of indices, we have that

1/xⁿ = x^-n

Differentiating both sides, we get that

$\dfrac{d}{dx} \big( \dfrac{1}{x^n}\big)$

= $\dfrac{d}{dx} \big( x^{-n} \big)$

= -n x^-n-1 by the power rule of derivatives

= $-\dfrac{n}{x^{n+1}}$.

So the derivative of 1 by x to the power n is equal to -n/xⁿ⁺¹, and this is obtained by the power rule of derivatives.

More Derivatives:

Derivative of xⁿ | Derivative of 1/x

Derivative of 1/x² | Derivative of ln(ln x)

FAQs