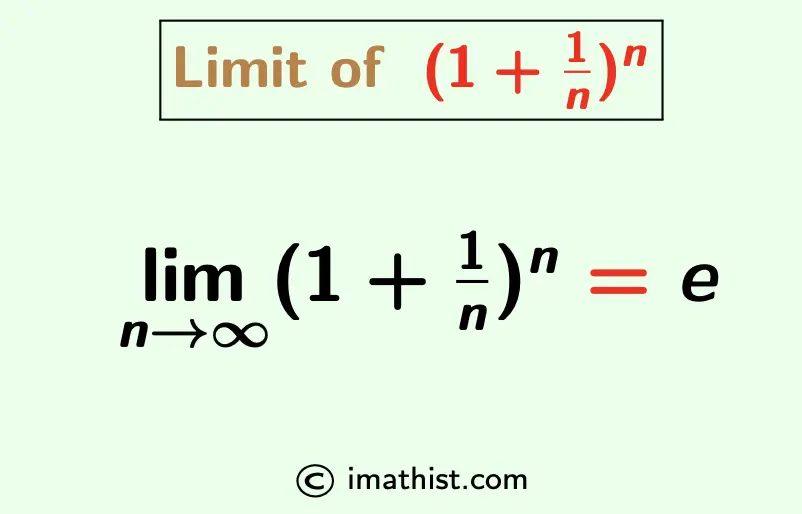
The limit of (1+1/n)^n as n approaches infinity is equal to e. The limn→∞ (1+1/n)n formula is given by
$\lim\limits_{n \to \infty} \big(1+\dfrac{1}{n} \big)^n=e$.
That is, the limit of (1+1/x)x when x→∞ is equal to e. In this post, we will learn to prove the limit of log(1+x)/x when x tends to zero.
Limn→∞ (1 + 1/n)n
Question: What is the limit of (1+1/n)n when n tends to infinity?
| Answer: The limit of (1+1/n)n is equal to e when n tends to infinity. |
Explanation:
Let the given limit is equal to y, that is,
$y=\lim\limits_{n \to \infty} \big(1+\dfrac{1}{n} \big)^n$.
We need to find the value of y. Taking natural logarithm ln=loge on both sides, we get that
$\ln y=\ln \lim\limits_{n \to \infty} \big(1+\dfrac{1}{n} \big)^n$.
⇒ $\ln y=\lim\limits_{n \to \infty} \ln \big(1+\dfrac{1}{n} \big)^n$ by the rule of limx→∞ ln(f(x)) = ln limx→∞ f(x).
⇒ $\ln y=\lim\limits_{n \to \infty}$ $\Big[ n \ln \big(1+\dfrac{1}{n} \big) \Big]$ using the logarithm rules
⇒ $\ln y=\lim\limits_{n \to \infty}$ $\Big[ n \big(\dfrac{1}{n}-\dfrac{1}{2n^2}+\dfrac{1}{3n^3}-\dfrac{1}{4n^4}+\cdots \big) \Big]$ using the formula of log(1+x).
⇒ $\ln y=\lim\limits_{n \to \infty}$ $\Big( 1-\dfrac{1}{2n}+\dfrac{1}{3n^2}-\dfrac{1}{4n^3}+\cdots \Big)$
⇒ $\ln y=1-0+0-0+\cdots $
⇒ $\ln y=1 =\ln e$
⇒ $y=e$
So the limit of (1+1/n)^n is equal to e when x→∞.
Read Also: Limit of (ex-1)/x when x→0
Remark: If we put n=x in the above limit formula, then we will get the limit of (1+1/x)x when x tends to infinity. Thus, the formula of limit of (1+1/x)x is equal to e when x→∞.
More Limits:
FAQs
Q1: What is the limit of (1+1/n)n when n tends to ∞?
Answer: The limit of (1+1/n)n when n tends to ∞ is equal to e, that is, limn→∞ (1+1/n)n =e.
Q2: What is the limit of (1+1/x)x when x tends to ∞?
Answer: The limit of (1+1/x)x when x tends to ∞ is equal to e, that is, limx→∞ (1+1/x)x =e.