Derivative of x^6: Proof by First Principle, Power Rule

The derivative of x^6 (x to the power 6) is equal to 6x⁵. Here, we will find the derivative of x⁶ using the power rule and the first principle of derivatives.

The derivative of x⁶ is denoted by d/dx (x⁶). The derivative formula of x⁶ is as follows:

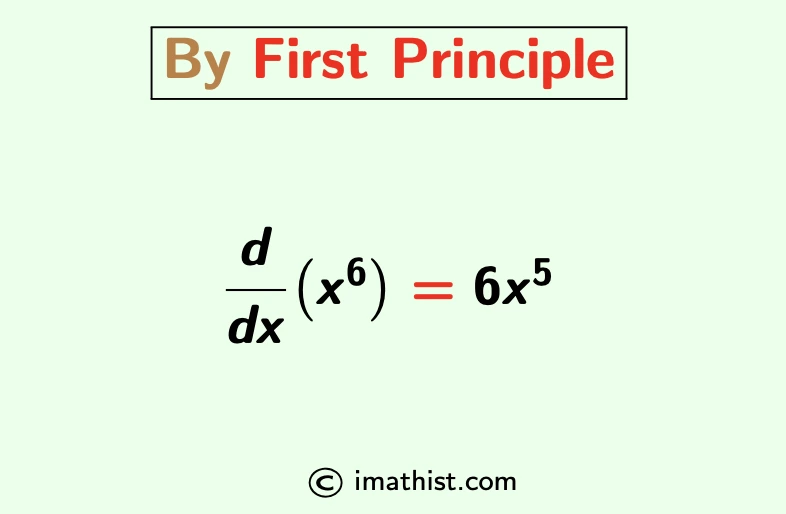
$\dfrac{d}{dx}(x^6)=6x^5$.

Derivative of x^6 by Power Rule

By the power rule of derivatives $\dfrac{d}{dx}(x^n)$ = nx^n-1, we get that

$\dfrac{d}{dx}(x^6)$ = 6x^6-1 = 6x⁵.

Hence the derivative of x⁶ by the power rule is 6x⁵.

You can Read: Derivative of xⁿ: Formula, Proof

Derivative of 10^x | Derivative of x¹⁰

Derivative of x^6 by First Principle

In first principle of derivatives formula $\dfrac{d}{dx}$(f(x)) = lim_h→0 $\dfrac{f(x+h)-f(x)}{h}$, we put

f(x)=x⁶.

So the derivative of x⁶ by first principle is given by

$\dfrac{d}{dx}(x^6)$ = lim_h→0 $\dfrac{(x+h)^6-x^6}{h}$

= lim_h→0 $\dfrac{(x+h)^6-x^6}{(x+h)-x}$

[Let x+h=z, so that z→x when h→0]

Thus, $\dfrac{d}{dx}(x^6)$

= lim_z→x $\dfrac{z^6-x^6}{z-x}$

= 6x^6-1 obtained by the formula lim_x→a $\dfrac{x^n-a^n}{x-a}$ = na^n-1.

= 6x⁵.

So the derivative of x^6 is 6x⁵ and this is proved by the first principle.

Also Read: Derivative of 2^x by first principle

Derivative of x⁴ by first principle

Derivative of e^sinx by first principle

FAQs