Cot(a+b) Cot(a-b) Formula, Proof

Cot(a+b) Cot(a-b) can be expressed in terms of cot as well as tan. In this post, we will learn to find cot(a+b)cot(a-b) formula with proof.

Let us now find the formula of the product cot(a+b) and cot(a-b).

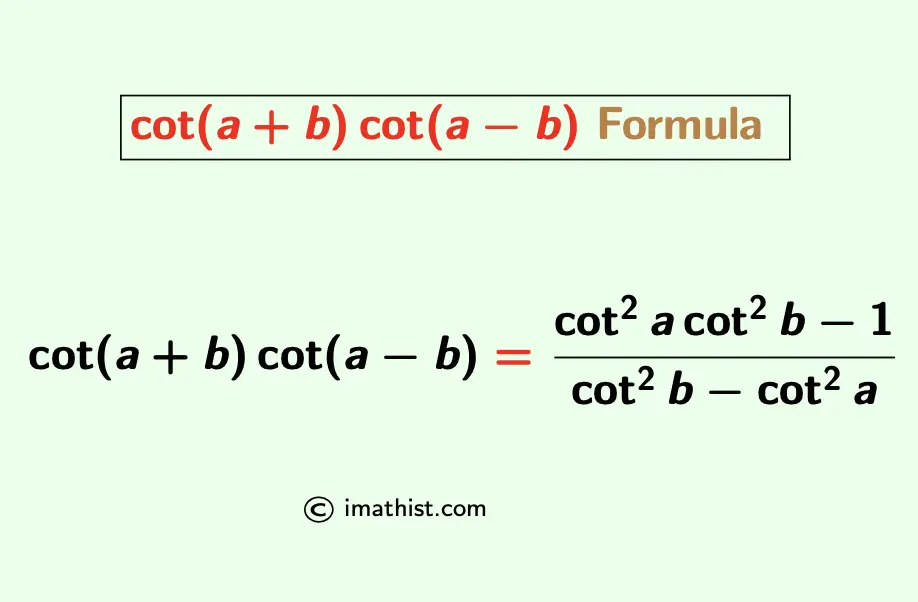
Cot(a+b) Cot(a-b) Formula in Terms of Cot

Using the identities of cot(a+b) and cot(a-b), the given expression can be written as follows:

cot(a+b) cot(a-b)

= $\dfrac{\cot a \cot b -1}{\cot b +\cot a} \times \dfrac{\cot a \cot b +1}{\cot b -\cot a}$

= $\dfrac{(\cot a \cot b -1)(\cot a \cot b +1)}{(\cot b +\cot a) (\cot b -\cot a)}$

= $\dfrac{(\cot a \cot b)^2 -1^2}{\cot^2 b -\cot^2 a}$, by the algebraic formula: (x-y)(x+y) =x²-y².

= $\dfrac{\cot^2 a \cot^2 b -1}{\cot^2 b -\cot^2 a}$

So cot(a+b) cot(a-b) formula in terms of cot is given by

cot(a+b) cot(a-b) = $\dfrac{\cot^2 a \cot^2 b -1}{\cot^2 b -\cot^2 a}$.

Also Read: Formula of sin(a+b) sin(a-b)

Formula of cos(a+b) cos(a-b)

cos(x+y+z) Formula, Proof

Cot(a+b) Cot(a-b) Formula in Terms of Tan

As cotx = $\frac{1}{\tan x}$, from the above formula we obtain that

cot(a+b) cot(a-b) = $\dfrac{\dfrac{1}{\tan^2 a \tan^2 b} -1}{\dfrac{1}{\tan^2 b} -\dfrac{1}{\tan^2 a}}$

Simplifying it we get that

cot(a+b) cot(a-b) = $\dfrac{1-\tan^2 a \tan^2 b}{\tan^2 a -\tan^2 b}$

So the formula of cot(a+b)cot(a-b) in terms of tan is given by cot(a+b)cot(a-b) = $\dfrac{1-\tan^2 a \tan^2 b}{\tan^2 a -\tan^2 b}$.

You can read: cosx cosy Formula, Proof

sinx siny Formula, Proof

sin(x+y+z) Formula, Proof

tan(x+y+z) Formula, Proof

FAQs