The derivative of sin(xy) is equal to (y+x dy/dx) cos(xy), and this is the derivative of sin(xy) with respect to x.
The derivative of sin(xy) formula is given below:
$\dfrac{d}{dx}(\sin xy)=(y+x\dfrac{dy}{dx})\cos xy$.
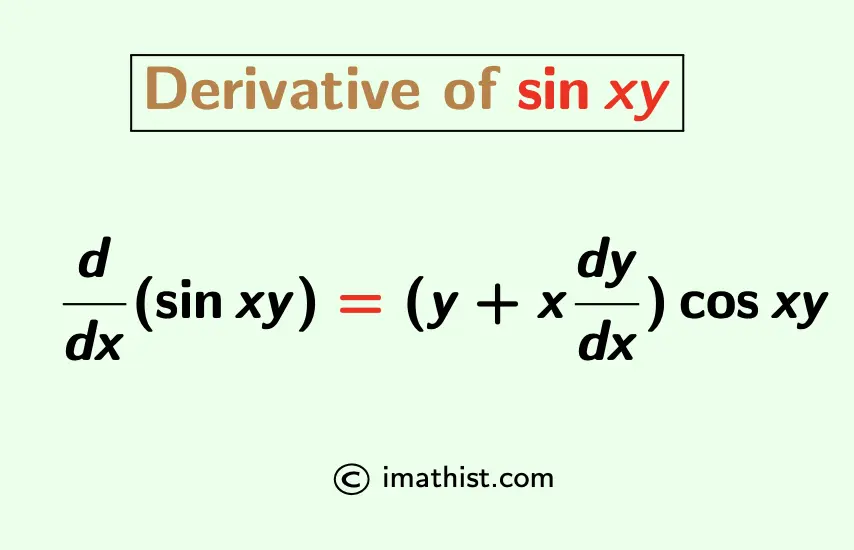
Differentiate sin(xy) with respect to x
Answer: The derivative of sin(xy) with respect to x is equal to (y+x dy/dx) cos(xy).
Explanation:
Let us use the chain rule of derivatives in order to differentiate sin(xy).
Put z=xy.
Differentiating with respect to x,
$\dfrac{dz}{dx}=y+x\dfrac{dy}{dx}$ by the product rule.
Now, by the chain rule,
$\dfrac{d}{dx}(\sin xy)= \dfrac{d}{dx}(\sin z)$
= $\dfrac{d}{dz}(\sin z) \times \dfrac{dz}{dx}$, by the chain rule.
= $\cos z \times (y+x\dfrac{dy}{dx})$ as the derivative of sinx is cosx.
= $\cos(xy) (y+x\dfrac{dy}{dx})$ as z=xy.
So the derivative of sin(xy) is equal to (y+x dy/dx) cos(xy), and this is obtained by the chain and product rule of derivatives.
FAQs
Q1: What is the derivative of sin(xy)?
Answer: The derivative of sin(xy) is (y+x dy/dx) cos(xy).