Derivative of 3^x by First Principle

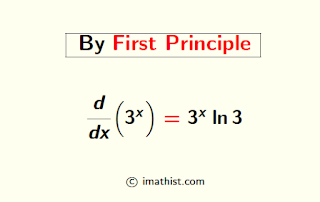
The derivative of 3^x is equal to 3^x ln 3. Here ln 3 denotes the natural logarithm of 3, that is, ln 3 = log_e 3. In this post, we will find the derivative of 3 to the power x by the first principle of derivatives.

We first recall the first principle of derivatives. The derivative of a function f(x) by first principle is given by the limit below:

d/dx (f(x)) = lim_h→0 $\dfrac{f(x+h)-f(x)}{h}$ …(I)

Derivative of 3^x from First Principle

To find the derivative of 3 to the x, we put f(x)=3^x in the above formula (I). Then by the first principle, the derivative of 3^x is given by

$\dfrac{d}{dx}$(3^x) = lim_h→0 $\dfrac{3^{x+h}-3^x}{h}$

= lim_h→0 $\dfrac{3^x \cdot 3^h -3^x}{h}$, by the indices rule: a^m+n = a^m ⋅ aⁿ

= lim_h→0 $\dfrac{3^x (3^h -1)}{h}$

= 3^x lim_h→0 $\dfrac{3^h -1}{h}$

= 3^x log_e3, here we have used the limit formula: lim_t→0 (a^t-1)/t = log_e a.

= 3^x ln 3

Thus, the derivative of 3^x is equal to 3^x ln 3 and this is obtained by the first principle of derivatives.

Note: From the derivative formula of a^x, we can obtain the derivative of 3^x. We know that $\dfrac{d}{dx}(a^x)=a^x \ln a$. Thus, the derivative of 3^x is equal to $\dfrac{d}{dx}(3^x)$ = 3^x ln 3.

Question: Find the derivative of 3^2x. That is, find $\dfrac{d}{dx}(3^{2x})$.

Answer:

Let z=2x. Then we have $\dfrac{dz}{dx}=2$. We will use the chain rule of derivatives. Thus, the derivative of 3^2x is given by

$\dfrac{d}{dx}(3^{2x})$ $=\dfrac{d}{dz}(3^z) \cdot \dfrac{dz}{dx}$

= 3^z ln3 × 2, as we have from the above that $\dfrac{d}{dx}$(3^x)=3^x ln 3.

= 2 ⋅ 3^2x ln 3, as we know z=2x.

Thus, the derivative of 3^2x is equal to 2⋅ 3^2x ln 3.

Also Read: 

Derivative of (sinx)^logx

Derivative of x^3/2

Derivative of 10^x

Integration of 1/(1+x²)

Derivative of 1/(1+x²)

FAQs