cosx siny Formula | cosx siny Identity

The function cosx siny is the product of a cosine function and a sine function. In this post, we will learn how to prove the formula/identity of cosx siny.

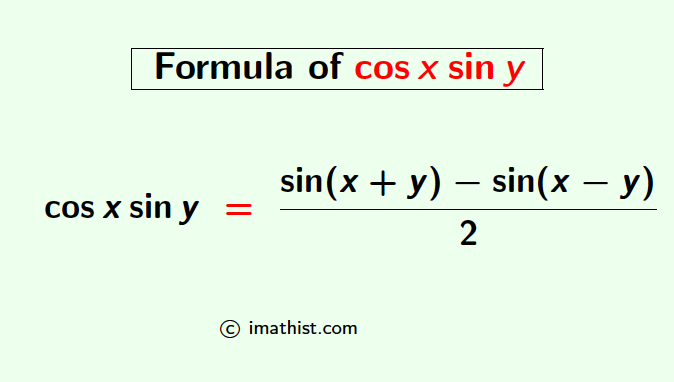
cosx siny Formula

The cosx siny formula is given as follows:

cosx siny = $\dfrac{\sin(x+y)-\sin(x-y)}{2}$

Let us now prove the above formula of cos x sin y.

Proof of cosx siny Formula

cosx siny = 1/2[sin(x+y) – sin(x-y)]

Proof:

Step 1: At first, we will write down the formulas of sin(x+y) and sin(x-y).

sin(x+y) = sinx cosy + cosx siny …(I)

sin(x-y) = sinx cosy – cosx siny …(II)

Step 2: Now, we will subtract (II) from (I). So we will get that

sin(x+y) – sin(x-y) = (sinx cosy + cosx siny) – (sinx cosy – cosx siny)

⇒ sin(x+y) – sin(x-y) = sinx cosy + cosx siny – sinx cosy + cosx siny

⇒ sin(x+y) – sin(x-y) = 2 cosx siny

Step 3: Lastly, we divide both sides by 2 to get the desired formula. By doing so, we deduce that

cosx siny = 1/2[sin(x+y) – sin(x-y)]

Thus we have obtain the formula of the product cosx siny which is cosx siny = 1/2[sin(x+y) – sin(x-y)].

From the above formula, we obtain the formula of 2cosx siny which is written below:

2cosx siny Formula: 2cosx siny = sin(x+y) – sin(x-y)

ALSO READ:

sinx cosy Formula

sinx siny Formula

cosx cosy Formula

Example 1:

Find the value of cos45 sin15.

Answer:

Putting x=45 and y=15 in the above formula of cosx siny, we obtain that

cos45 sin15 = $\dfrac{\sin(45+15)-\sin(45-15)}{2}$

= $\dfrac{\sin 60-\sin 30}{2}$

= $\dfrac{\frac{\sqrt{3}}{2} -\frac{1}{2}}{2}$

= $\dfrac{\frac{\sqrt{3}-1}{2}}{2}$

= $\dfrac{\sqrt{3}-1}{4}$

So the value of cos45 sin15 is equal to (√3-1)/4 and this is obtained by applying the formula of cosx siny.

FAQs