Derivative of e^sinx by First Principle

The derivative of e^sinx is equal to cosx e^sinx. In this post, we will find the derivative of e^sinx by first principle.

The e^sinx derivative formula is given as follows:

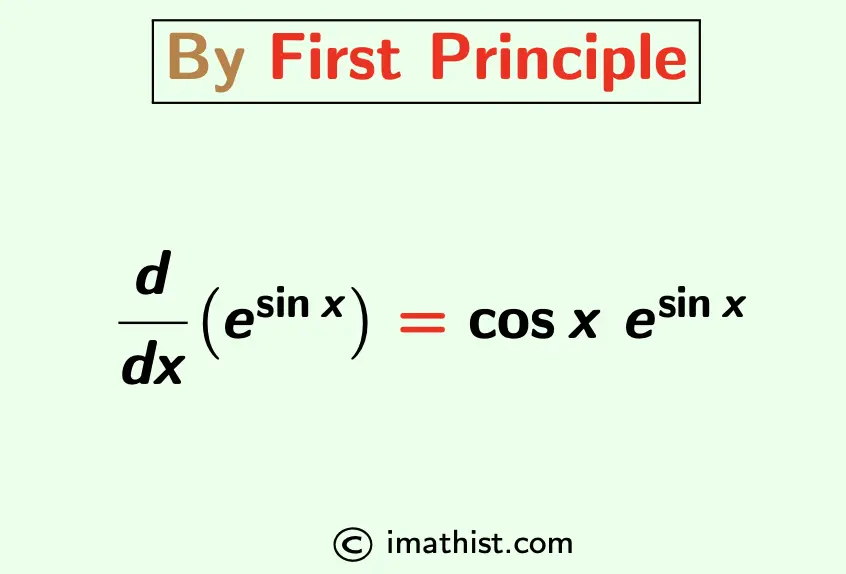
$\dfrac{d}{dx}$(e^sinx) = cosx e^sinx.

Derivative of e^sinx Using First Principle

The derivative of f(x) using the first principle is given by the following limit formula:

$\dfrac{d}{dx}$ ( f(x) ) = lim_h→0 $\dfrac{f(x+h)-f(x)}{h}$.

Put f(x) = e^sinx.

So $\dfrac{d}{dx}$ ( e^sinx ) = lim_h→0 $\dfrac{e^{\sin(x+h)} – e^{\sin x}}{h}$

= lim_h→0 $\dfrac{e^{\sin x}(e^{\sin(x+h)-\sin x} -1)}{h}$

= e^sinx lim_h→0 $\dfrac{e^{\sin(x+h)-\sin x} -1}{h}$

= e^sinx lim_h→0 $\dfrac{e^{\sin(x+h)-\sin x} -1}{\sin(x+h)-\sin x}$ × lim_h→0 $\dfrac{\sin(x+h)-\sin x}{h}$

[Let z=sin(x+h)-sin x. Then z→0 as h→0]

= e^sinx lim_z→0 $\dfrac{e^z-1}{z}$ × lim_h→0 $\dfrac{2\cos(x+\frac{h}{2})\sin \frac{h}{2}}{h}$, using the formula sina – sinb = 2 cos(a+b)/2 sin(a-b)/2.

= e^sinx × 1 × lim_h→0 $\cos(x+\frac{h}{2})$ × lim_h→0 $\dfrac{\sin \frac{h}{2}}{\frac{h}{2}}$ as the limit lim_x→0(e^x-1)/x =1.

= e^sinx cos(x+\frac{0}{2}) × lim_u→0 sinu/u where u=h/2.

= e^sinx cosx × 1

= cosx e^sinx.

So the derivative of e^sinx is equal to cosx e^sinx, and this is obtained by the first principle of derivatives.

More Derivatives:

Derivative of 1/lnx

Derivative of ln (lnx)

Derivative of root sinx

Derivative of root cosx

Derivative of mod x

FAQs