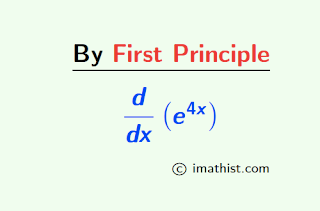
The derivative of e^4x with respect to x is 4e^4x. In this post, we will evaluate the derivative of e to the power 4x from first principle.
Derivative of e^4x by first principle
Let f(x) be a function in one variable x. The derivative of f(x) from first principle is given by the following limit:
`d/dx(f(x))` `=lim_{h to 0} frac{f(x+h)-f(x)}{h}` `quad cdots` (i)
Take `f(x)=e^{4x}` in the above formula (i). So the derivative of `e^{4x}` by first principle is equal to
`d/dx(e^{4x})` `=lim_{h to 0} frac{e^{4(x+h)}-e^{4x}}{h}`
`=lim_{h to 0} frac{e^{4x+4h}-e^{4x}}{h}`
`=lim_{h to 0} frac{e^{4x} cdot e^{4h}-e^{4x}}{h}` using the rule of indices `e^{a+b}` `=e^acdot e^b`
`=lim_{h to 0} frac{e^{4x}(e^{4h}-1)}{h}`
`=e^{4x} lim_{h to 0} frac{e^{4h}-1}{h}` (As the function `e^{4x}` is independent of h. So one can take it out of the limit)
`=e^{4x} lim_{h to 0} frac{e^{4h}-1}{4h}` `times 4`
Let `4h=t`. So `t to 0` as h tends to zero. So the above limit is
`=4e^{4x} lim_{t to 0} frac{e^t-1}{t}`
`=4e^{4x} times 1` using the limit formula `lim_{x to 0} frac{e^{x}-1}{x}=1`
`=4e^{4x}`
Thus the derivative of e to the power 4x is 4e^4x and this is obtained by the first principle of derivatives.
Question Answer on Derivative of e^4x
Question 1: Find the derivative of e^4x at x=0 from first principle.
Answer:
The derivative of e^4x from the first principle is `4e^{4x}` (see the above proof).
Thus, the derivative of e^4x at x=0 is
`[d/dx(e^{4x})]_{x=0}` `=[4e^{4x}]_{x=0}` `=4e^{4cdot 0}` `=4e^0` `=4 times 1` `=4`
Hence the derivative of e^4x at x=0 by the first principle is equal to 4.
Question 2: Find the derivative of e^4.
Answer:
Note that `e^4` is a constant number as the number e is so. We know that the derivative of a constant is zero (For a proof of this fact, click the page on Derivative of a constant is 0). So we conclude that the derivative of e to the power 4 is zero.
Also Read: