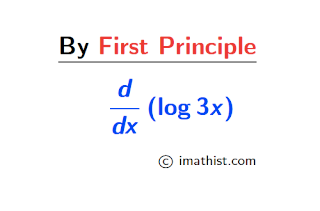
The derivative of log3x is 1/x. In this post, we will find the derivative of log 3x by first principle. To do so, we will use the following limit formula on logarithm functions:
$\lim\limits_{x \to 0} \dfrac{\log(1+x)}{x}=1$ $\quad \cdots (i)$
Derivative of log 3x from First Principle
The first principle of derivatives says that the derivative of a function f(x) is given by the following limit:
$\dfrac{d}{dx}(f(x))$ $=\lim\limits_{h \to 0} \dfrac{f(x+h)-f(x)}{h}$ $\quad \cdots (ii)$
To find the derivative of log 3x using first principle, let us assume that
$f(x)=\log 3x$
Then from (ii) we get that
$\dfrac{d}{dx}(\log 3x)$ $=\lim\limits_{h \to 0} \dfrac{\log 3(x+h)-\log 3x}{h}$
$=\lim\limits_{h \to 0} \dfrac{\log (3x+3h)-\log 3x}{h}$
Using the formula $\log a -\log b$ $=\log \frac{a}{b}$ the above is
$=\lim\limits_{h \to 0} \dfrac{\log \frac{3x+3h}{3x}}{h}$
$=\lim\limits_{h \to 0} \dfrac{\log (1+\frac{h}{x})}{h}$
The above can be rewritten as
$=\lim\limits_{h \to 0} \dfrac{\log (1+\frac{h}{x})}{h/x}$ $\times \dfrac{1}{x}$
Let $t=\dfrac{h}{x}$. Then $t \to 0$ as h tends to zero.
So the derivative of log 3x is
$\dfrac{d}{dx}(\log 3x)$ $=\lim\limits_{t \to 0} \dfrac{\log (1+t)}{t}$ $\times \dfrac{1}{x}$
$=1 \times \dfrac{1}{x}$ by the above limit formula (i)
$=\dfrac{1}{x}$
Thus the derivative of log 3x is 1/x and this is obtained by the first principle of derivatives.
Question: Find the derivative of log 3x at x=1 by first principle.
Answer:
From the above, we know that the derivative of log 3x by the first principle is 1/x, that is,
$\dfrac{d}{dx}(\log 3x)=\dfrac{1}{x}$
So the derivative of log 3x at x=1 will be
$[\dfrac{d}{dx}(\log 3x)]{x=1}=\dfrac{1}{x}|_{x=1}$ $=1/1$ $=1$
Also Read:
Derivative of e^3x from first principle
Derivative of log(sin x) from first principle
Derivative of root(1+x) from first principle
Derivative of log(cos x) from first principle
Derivative of root sinx from first principle
Derivative of root cosx from first principle
FAQs
Q1: What is the derivative of log3x?
Answer: The derivative of log3x is 1/x if the logarithm base is e.