Derivative of Natural log of x: Formula, Proof

The derivative of natural log of x is equal to 1/x. As the natural logarithm of x is denoted by lnx, so the derivative formula of natural log of x is given by

$\dfrac{d}{dx}{\ln x}=\dfrac{1}{x}$.

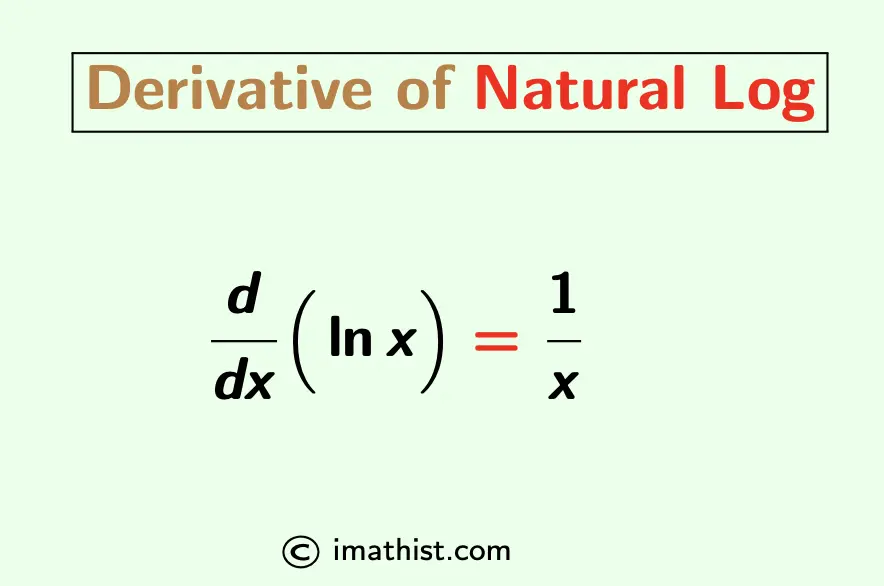
Find Derivative of Natural log of x

Answer: The derivative of natural log of x is 1/x.

Explanation:

Note the natural log of x is denoted by ln(x) = log_ex. Let us assume that

y= ln(x) = log_ex. (we need to find dy/dx)

⇒ e^y = x.

Differentiating both sides with respect to x, we get that

e^y $\dfrac{dy}{dx}$ = 1

⇒ $\dfrac{dy}{dx} = \dfrac{1}{e^y}$

⇒ $\dfrac{dy}{dx}$ = e^-y

⇒ $\dfrac{dy}{dx}$ = e^{-ln x} = $e^{\ln x^{-1}}$

⇒ $\dfrac{dy}{dx}$ = x^-1 = 1/x.

So the derivative of natural log of x is equal to 1/x, and this is obtained by the method of implicit differentiation.

More Derivatives: Derivative of a^x by first principle

Derivative of e^sinx by first principle

Derivative of root tanx by first principle

Derivative of sec2x by first principle

Derivative of Natural log of x by First Principle

By first principle, the derivative of f(x) is given by

$\dfrac{d}{dx}$(f(x)) = lim_h→0 $\dfrac{f(x+h)-f(x)}{h}$.

Put f(x) = ln(x).

So the derivative of ln(x), natural log of x, is given as follows:

$\dfrac{d}{dx}$( ln(x) ) = lim_h→0 $\dfrac{\ln(x+h)-\ln(x)}{h}$

= lim_h→0 $\dfrac{\ln(\dfrac{x+h}{x})}{h}$ by the formula: lna -lnb = ln(a/b).

= lim_h→0 $\dfrac{\ln(1+\frac{h}{x})}{h}$

= $\dfrac{1}{x}$ lim_h→0 $\dfrac{\ln(1+\frac{h}{x})}{h/x}$

[Let h/x=t, so t→0 as h→0]

= $\dfrac{1}{x}$ lim_t→0 $\dfrac{\ln(1+t)}{t}$

= $\dfrac{1}{x} \times 1$

= $\dfrac{1}{x}$.

So the derivative of natural log of x by the first principle is equal to 1/x.

Read Also: Derivative of e^cosx by first principle

Derivative of $\sqrt{\sin x}$ by first principle

Derivative of $\sqrt{\cos x}$ by first principle

FAQs