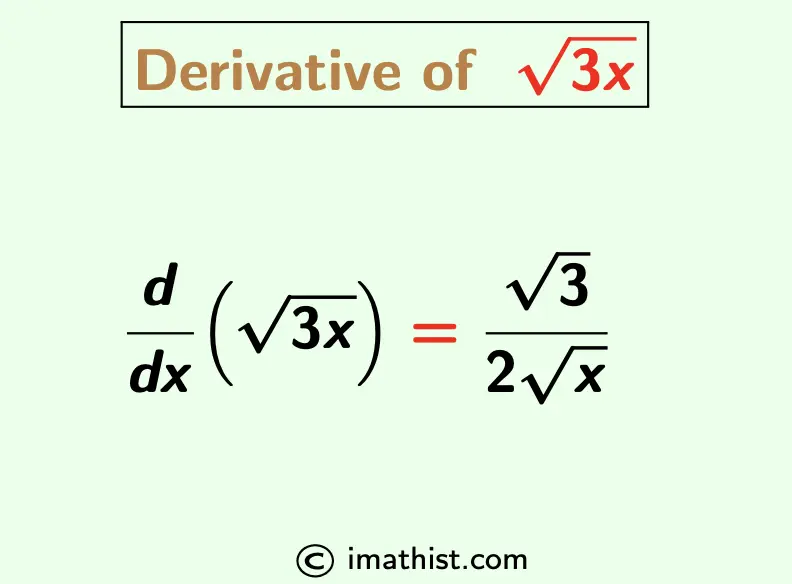
Derivative of root 3x | Root 3x Derivative

The derivative of root 3x is equal to √3/(2√x). In this post, we will learn how to find the derivative of the square root of 3x.

Now, we find the derivative of square root of 3x by the power rule.

Table of Contents

Derivative of root 3x by Power Rule

Let us find the derivative of square root of 3x by the power rule of derivatives. The rule says that the derivative of xⁿ is given by the formula:

$\frac{d}{dx}$(xⁿ) = nx^n-1.

Now, $\dfrac{d}{dx}(\sqrt{3x})$

= √3 × $\dfrac{d}{dx}(\sqrt{x})$

= √3 × $\dfrac{d}{dx}(x^{1/2})$ as we know that square root of x is written as x to the 1/2.

= $\sqrt{3} \times \dfrac{1}{2}x^{1/2 -1}$ by the above power rule.

= $\dfrac{\sqrt{3}}{2}x^{-1/2}$

= $\dfrac{\sqrt{3}}{2\sqrt{x}}$.

So the derivative of square root 3x is √3/(2√x), and this is obtained by using the power rule of derivatives.

Next, we find the derivative of root 3x by the chain rule of derivatives.

Let z=3x.

So dz/dx =3.

By the chain rule, the derivative of root 3x will be equal to

$\dfrac{d}{dx}(\sqrt{3x})$

= $\dfrac{d}{dz}(\sqrt{z})$ × $\dfrac{dz}{dx}$

= $\dfrac{1}{2\sqrt{z}}$ × $\dfrac{dz}{dx}$ as the derivative of root x is 1/(2√x).

= $\dfrac{1}{2\sqrt{3x}}$ × 3 as z=3x.

= √3/(2√x)

So the derivative of square root 3x by chain rule is √3/(2√x).

Have You Read These?

Answer: The derivative of the square root of 3x is √3/(2√x).

Spread the love