Find Derivative of x/y [x divided by y]

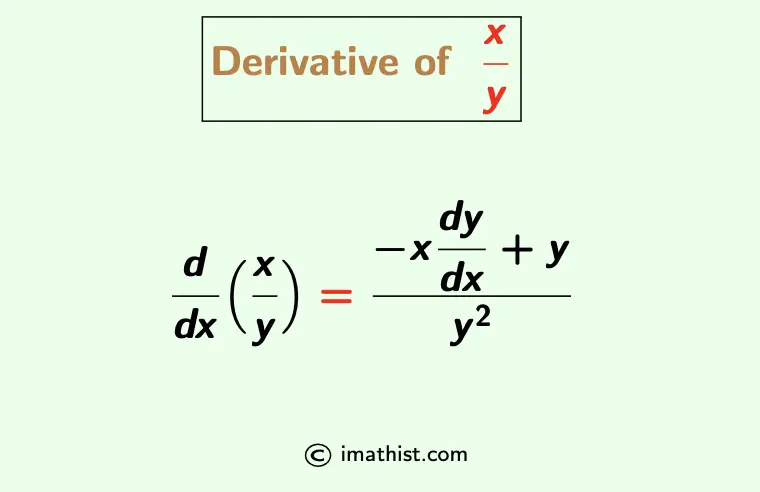
The derivative of x/y with respect to x is equal to (-x dy/dx + y)/y². Here, we will learn how to differentiate x/y with respect to x.

Derivative of x/y with respect to x

Question: Differentiate x/y with respect to x.

Solution:

Note that $\dfrac{x}{y}$ is a product of two functions $x$ and $\dfrac{1}{y}$. So to find its derivative with respect to $x$, we will use the product rule of derivatives.

We have

$\dfrac{d}{dx} \big( \dfrac{x}{y}\big)$ $=\dfrac{d}{dx} \big( x \cdot \dfrac{1}{y}\big)$

= $x \cdot \dfrac{d}{dx} \big(\dfrac{1}{y}\big) + \dfrac{1}{y} \cdot \dfrac{d}{dx} (x)$

= $x \cdot \dfrac{d}{dx} (y^{-1}) + \dfrac{1}{y} \cdot 1$

= $x \cdot (-1 \cdot y^{-1-1})\dfrac{dy}{dx} + \dfrac{1}{y}$, by the power rule and the chain rule of derivatives.

= $-x \cdot y^{-2}\dfrac{dy}{dx} + \dfrac{1}{y}$

= $-\dfrac{x}{ y^2} \dfrac{dy}{dx} + \dfrac{1}{y}$

= $\dfrac{-x \dfrac{dy}{dx}+y}{ y^2}$

So the differentiation of x/y with respect to x is equal to (-x dy/dx + y)/y².

Derivative of x/y with respect to y

Question: Differentiate x/y with respect to y.

Solution:

In a similar way as above, we have that

$\dfrac{d}{dy} \big( \dfrac{x}{y}\big)$ $=\dfrac{d}{dy} \big( x \cdot \dfrac{1}{y}\big)$

= $x \cdot \dfrac{d}{dy} \big(\dfrac{1}{y}\big) + \dfrac{1}{y} \cdot \dfrac{d}{dy} (x)$

= $x \cdot \dfrac{d}{dy} (y^{-1}) + \dfrac{1}{y} \dfrac{dx}{dy}$

= $x \cdot (-1 \cdot y^{-1-1})+ \dfrac{1}{y}\dfrac{dx}{dy}$, by the power rule of derivatives.

= $\dfrac{-x}{y^2}+ \dfrac{1}{y}\dfrac{dx}{dy}$

= $\dfrac{-x+y \frac{dx}{dy}}{y^2}$

Hence the differentiation of x/y with respect to y is equal to (-x+ y dx/dy)/y².

Also Read: Derivative of Square Root of 2x

FAQs