The derivative of x^10 (x to the power 10) is 10x9. In this post, we will find the derivative of x10 by the first principle and the power rule of derivatives.
The derivative of x10 is denoted by d/dx (x10), and its formula is given by
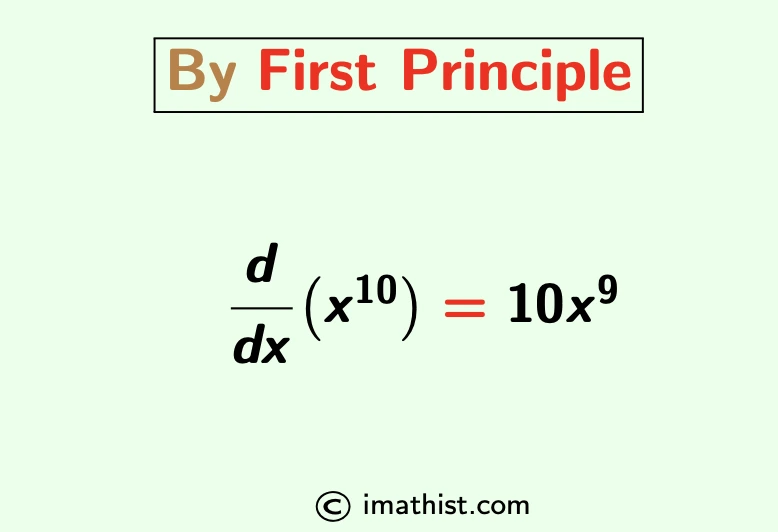
$\dfrac{d}{dx}(x^{10})=10x^9$.
Derivative of x^10 by First Principle
The derivative of a function f(x) by the first principle is equal to the limit $\dfrac{d}{dx}$(f(x)) = limh→0 $\dfrac{f(x+h)-f(x)}{h}$.
Let us Put f(x)=x10.
Hence the derivative of x10 using the first principle is equal to
$\dfrac{d}{dx}(x^{10})$ = limh→0 $\dfrac{(x+h)^{10}-x^{10}}{h}$
= limh→0 $\dfrac{(x+h)^{10}-x^{10}}{(x+h)-x}$
[Let x+h=t, so that t→x when h→0]
Thus, $\dfrac{d}{dx}(x^{10})$
= limt→x $\dfrac{t^{10}-x^{10}}{t-x}$
= 10x10-1 by the limit formula: limx→a $\dfrac{x^n-a^n}{x-a}$ = nan-1.
= 10x9.
So the derivative of x^10 by the first principle is equal to 10x9.
Also Read: Derivative of x4 by first principle
Derivative of x5 by first principle
Derivative of x3/2 | Derivative of 2x
Derivative of esinx by first principle
Derivative of ecosx by first principle
Derivative of x^10 by Power Rule
In the power rule of derivatives $\dfrac{d}{dx}(x^n)$ = nxn-1, we put
n=10.
Thus, $\dfrac{d}{dx}(x^{10})$ = 10x10-1 = 10x9.
So the derivative of x^10 by the power rule is 10x9.
Related Topics: Derivative of xn: Formula, Proof
Question-Answer
We now find the derivative of e to the power 10x.
Question: Find the derivative of e10x.
Answer:
Let z=ex. So dz/dx = ex.
By the chain rule of derivatives,
$\dfrac{d}{dx}(e^{10x})=\dfrac{d}{dx}(z^{10})$
= $\dfrac{d}{dz}(z^{10}) \cdot \dfrac{dz}{dx}$
= $10z^{10-1} \cdot e^x$
= 10 e9x ⋅ ex as z=ex
= 10 e10x.
So the derivative of e^{10x} is equal to 10 e10x.
ALSO READ: Derivative of 1/ln(x)
Derivative of e3x | Derivative of xe-x
FAQs
Q1: What is the derivative of x10?
Answer: The derivative of x10 is equal to 10x9.