Find the Values of sin18, cos18

The value of sin18° is equal to (√5-1)/4 and the value of cos18° is equal to √(10+2√5)/2. Here we will learn to find the values of sin18, cos18.

The sin 18 degree formula is given by

$\boxed{{\displaystyle \sin {18}^{\circ }={\displaystyle \frac{\sqrt{5}-1}{2}}}}$

The cos 18 degree formula is given by

$\boxed{{\displaystyle \cos {18}^{\circ }={\displaystyle \frac{\sqrt{10+2\sqrt{5}}}{2}}}}$

Value of sin18

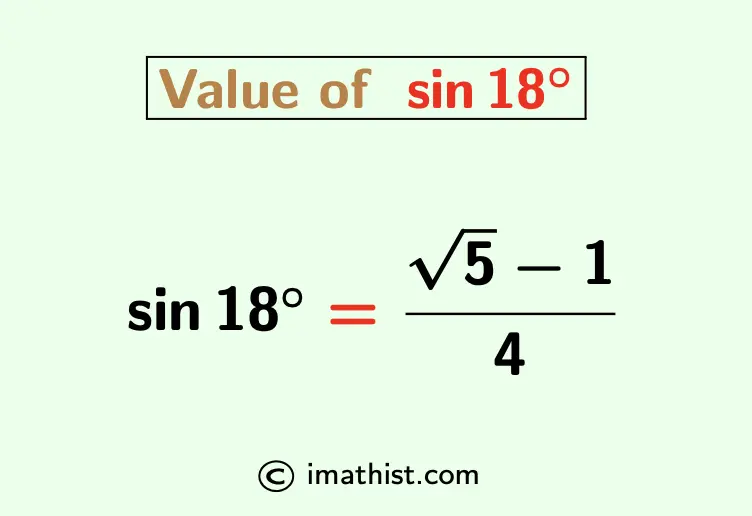
Question: Find the value of sin18°.

Answer:

Let us assume

θ = 18°

[We need to find sinθ]

⇒ 5θ = 90°

⇒ 3θ+2θ = 90°

⇒ 2θ = 90° -3θ

Taking sin on both sides, we get that

sin2θ = sin(90° -3θ)

⇒ sin2θ = cos3θ, by the formula sin(90-x)=cosx.

⇒ 2sinθ cosθ = 4cos³θ -3cosθ, using the formula sin2A= 2sinA cosA, and cos3A= 4cos³A -3cosA.

⇒ 2 sinθ cosθ – 4cos³θ + 3cosθ = 0

⇒ cosθ (2sinθ – 4cos²θ + 3) = 0

As cosθ = cos18° ≠ 0, we get that

2sinθ – 4cos²θ + 3 =0

⇒ 2sinθ – 4(1-sin²θ) + 3 =0 as sin²θ+cos²θ =1.

⇒ 4sin²θ + 2sinθ – 1 =0

So sin18° is a positive root of the quadratic equation 4x²+2x-1=0.

By the rule of quadratic equations,

sinθ = ${\displaystyle \frac{-2\pm \sqrt{4+16}}{2\times 4}}$ = ${\displaystyle \frac{-1\pm \sqrt{5}}{4}}$

As sinθ = sin18° lies in the first quadrant, its value is positive. In other words, we conclude that

sinθ = ${\displaystyle \frac{-1+\sqrt{5}}{4}}$.

Thus, the value of sin18° is equal to (√5-1)/4.

Also Read: Values of sin15, cos 15, tan15

Values of sin75, cos75, tan75

Value of cos18

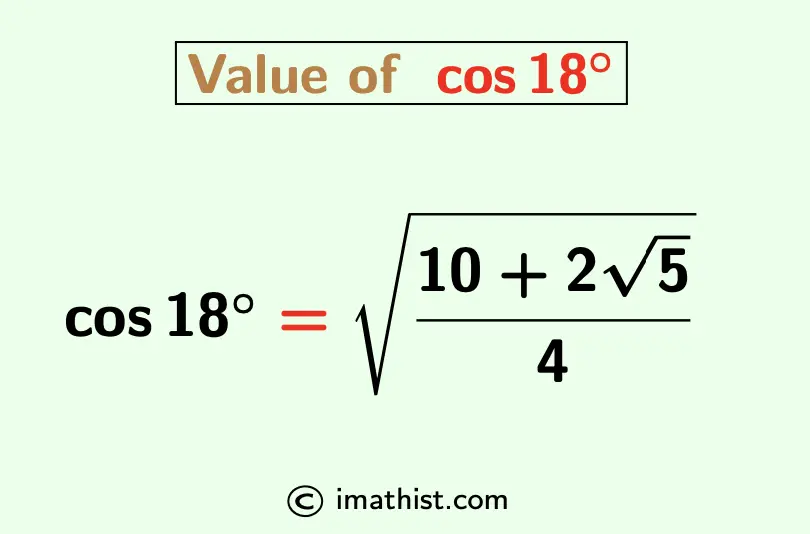
Question: Find the value of cos18°.

Answer:

Let θ = 18°

Using the identity sin²θ + cos²θ =1, we have that

cos18° = cosθ = $\sqrt{1-{\sin }^2\theta }$

⇒ cos18° = $\sqrt{1-{\sin }^2{18}^{\circ }}$

⇒ cos18° = $\sqrt{1-({\displaystyle \frac{\sqrt{5}-1}{2}}{)}^2}$ as sin18° = (√5-1)/4 by above.

= $\sqrt{{\displaystyle \frac{16-5+2\sqrt{5}-1}{4}}}$

= $\sqrt{{\displaystyle \frac{10+2\sqrt{5}}{4}}}$

So the value of cos18° is equal to $\sqrt{{\displaystyle \frac{10+2\sqrt{5}}{4}}}$.

Also Read: Sin3x in terms of sinx

FAQs