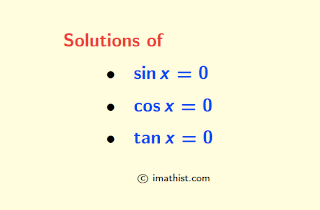General Solution of sin x =0, cos x=0, tan x=0

One can define equations involving trigonometric functions. These equations are called trigonometric equations. In this post, we will learn about the general solutions of the trigonometric equations: sin x=0, cos x =0, and tan x =0.

Solution of sin x =0

Solve $\sin x =0$.

The general solutions of the equation $\sin x =0$ are the integer multiples of $\pi$. In other words:

$\sin x =0$ if and only if $x=n \pi$ where n is an integer.

So the solutions of sin x =0 are x=nπ where n is an integer.

Solution of cos x =0

Solve $\cos x =0$.

The general solutions of the equation $\cos x =0$ are the odd multiples of $\dfrac{\pi}{2}$. In other words:

$\cos x =0$ if and only if $x=(2n+1) \dfrac{\pi}{2}$ where some integer n.

Thus the solutions of cos x =0 are x=(2n+1)$\dfrac{\pi}{2}$ where n is an integer.

Solution of tan x =0

Solve tan x =0.

Note that $\tan x =0$

$\Rightarrow \dfrac{\sin x}{\cos x}=0$

$\Rightarrow \sin x=0$

Therefore, the general solutions of the equation $\tan x =0$ are given by the solutions of the equation $\sin x =0$.

From above we know that $\sin x =0$  $\iff x=n \pi$ where $n \in Z$. So the general solutions of $\tan x =0$ are the integer multiples of $\pi$. In other words:

$\tan x =0$ if and only if $x=n \pi$ where n is an integer.

Hence the solutions of tan x =0 are x=n$\pi$ where n is an integer.

Example1: Solve sin 2x =0.

Solution:

As the solutions of sin x =0 are x=n$\pi$, we deduce that the solutions of sin 2x =0 are

$2x=n \pi$

$\Rightarrow x =\dfrac{n\pi}{2}$

So the solutions of sin 2x =0 are $x =\dfrac{n\pi}{2}$ where n is an integer.

Example2: Solve sin 3x =0.

Solution:

We know that the solutions of sin x =0 are x=n$\pi$. Thus the solutions of sin 3x =0 are

$3x=n \pi$

$\Rightarrow x =\dfrac{n\pi}{3}$

Thus the solutions of sin 3x =0 are $x =\dfrac{n\pi}{3}$ where n is an integer.

Example3: Solve cos 3x =0.

Solution:

We know that the solutions of cos x =0 are x=(2n+1)$\dfrac{\pi}{2}$. Thus the solutions of cos 3x =0 are

$3x=(2n+1) \dfrac{\pi}{2}$

$\Rightarrow x =(2n+1)\dfrac{\pi}{6}$

So the solutions of cos 3x =0 are $x =(2n+1)\dfrac{\pi}{6}$ where n is an integer.

ALSO READ:

Values of sin15, cos15, tan15

Values of sin75, cos75, tan75

Simplify sin(cos^-1x)

Sin3x formula in terms of sinx

FAQs