[Solved] What is the Integral of 0? | Integration of zero

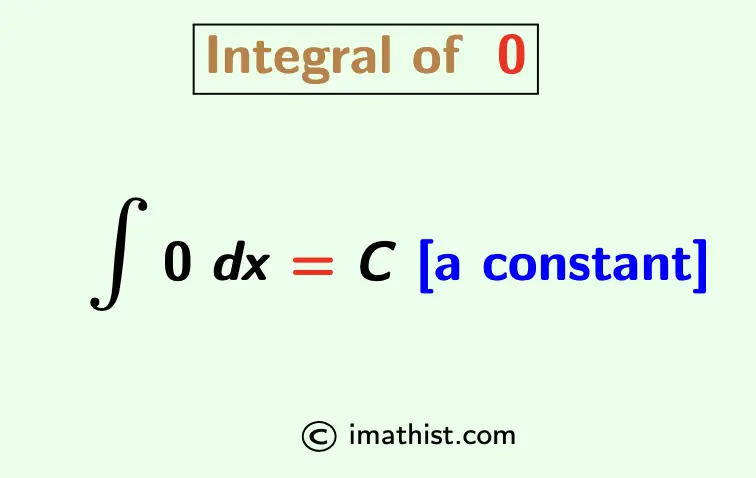
The integral of 0 is equal to C where C is an arbitrary constant. The integration of 0 is denoted by ∫0 dx, and its formula is given by

∫0 dx = C

where C is an integration constant. In this post, we will learn how to integrate the constant 0.

NOTE: We know that the derivative of a constant is 0. That is,

$\dfrac{d}{dx}$ (constant) = 0.

As the derivative is the opposite process of the integration, we conclude that the integral of zero is given by

∫0 dx = any constant C.

Integration of 0

Question: What is the integration of 0?

Solution:

Using the multiplication by constant rule of integration, we have

∫0 dx = 0 ∫dx + C where C is a constant

⇒ ∫0 dx = 0 + C

⇒ ∫0 dx = C.

So the integration of 0 (zero) is equal to C, a constant.

Read Also: Integral of 1/x²

What is the integration of x²

Definite Integral of 1

Question: Find the definite integral of 0 from -1 to 1, that is, Find ∫-11 0 dx.

Answer

From above, we know that the integral of 0 is a constant C. Thus,

$\int 0 \ dx$ $=\Big[C \Big]_{-1}^1$

= C-C

= 0

So the definite integration of 0 from -1 to 1 is equal to 0.

You Can Read: Integral of secx

Integration of tan x

FAQs