Integral of cos root x dx | Find ∫cos(√x)dx

The integral of cos root x dx is denoted by ∫cos(√x)dx, and it is equal to ∫cos(√x)dx = 2[√xsin(√x)+ cos(√x)]+C where C is an integration constant. Here we will learn how to integrate cos root x.

The integral formula of cos root x is given below.

$\int \cos \sqrt{x} dx$ $= 2[\sqrt{x} \sin \sqrt{x}+\cos \sqrt{x}]+C$

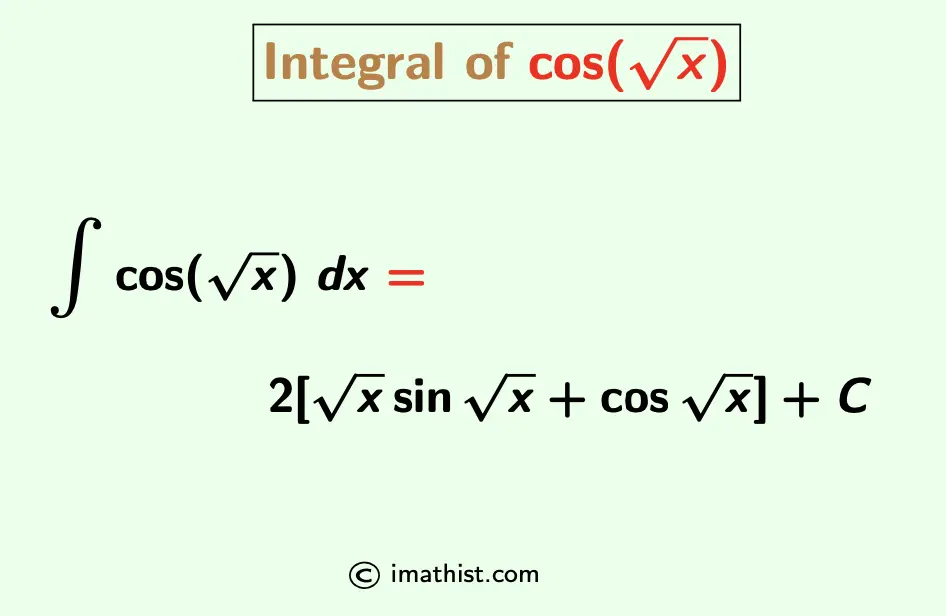
Integration of cos root x

Question: Find the integral ∫cos(√x)dx.

Answer:

Let us put

I = ∫cos(√x)dx.

Put √x=t, that is, x=t².

Differentiating both sides, we get

dx = 2tdt

So, I = ∫cos(√x)dx = 2 ∫tcos(t) dt

To compute this integral, we use the integration by parts formula: ∫uv dx = u ∫v dx – ∫[$\frac{du}{dx}$∫v dx] dx (where u, v are functions of x).

Take

u= t, v=cost.

Thus,

I = 2 ∫tcos(t) dt

= 2 [t ∫cost dt – ∫{$\frac{dt}{dt}$∫cost dt} dt ] + C

= 2[t sint – ∫sint dt] + C

= 2[t sint + cost] + C

= 2[√x sin(√x) + cos(√x)] + C.

So the integral of cos(root x) is equal to ∫cos(√x)dx = 2[√x sin(√x) + cos(√x)] + C where C is a constant.

More Integrals:

Integral of sin root x

Integration of root tanx + root cotx

Integral of square root of tanx

FAQs