Integral of e^(x^2) | Integration of e^(x^2)

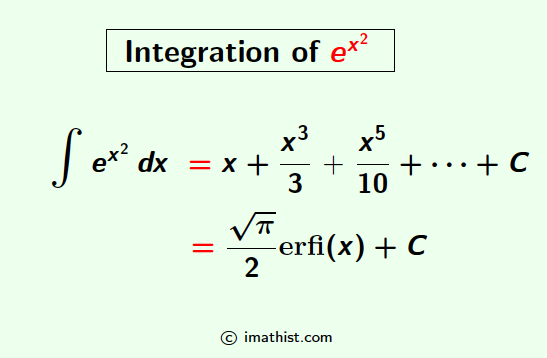
 The integral of e^(x^2), that is, the integration of e to the x² is equal to $\dfrac{\sqrt{\pi}}{2}$ erfi(x)+C, where erfi(x) is called the “imaginary error function” and C is an integration constant. In this post, we will learn to integrate the function $e^{x^2}$.

Integral of $e^{x^2}$

We know that e^x can be written as

e^x = $\sum_{n=0}^\infty \dfrac{x^n}{n!}$

= $1+x+\dfrac{x^2}{2!}+\dfrac{x^3}{3!}+\cdots +$ $\dfrac{x^n}{n!}+\cdots$

Thus, we can write $e^{x^2}$ as follows:

$e^{x^2}$ = $1+x^2+\dfrac{x^4}{2!}+\cdots +\dfrac{x^{2n}}{n!}+\cdots$

∴ $e^{x^2}$ = $1+x^2+\dfrac{x^4}{2}+\cdots +\dfrac{x^{2n}}{n!}+\cdots$

Now, to get the integration of e to the x², we will integrate term-by-term. By doing so, we will get that

$\int e^{x^2} \ dx$ = $\int 1 \ dx + \int x^2 \ dx$ $+\int \dfrac{x^4}{2} \ dx +\cdots +\int \dfrac{x^{2n}}{n!} \ dx +\cdots$

= x + $\dfrac{x^3}{3}$ + $\dfrac{x^5}{10} + \cdots $ $+\dfrac{x^{2n+1}}{(2n+1) n!}+\cdots$

So integrating term-by-term we obtain the integration of e to the x² which is equal to x + $\dfrac{x^3}{3}$ + $\dfrac{x^5}{10} + \cdots $ $+\dfrac{x^{2n+1}}{(2n+1) n!}+\cdots+C$, where C is an integration constant. This function is known as $\dfrac{\sqrt{\pi}}{2}$ erfi(x)+C where erfi(x) is the “imaginary error function” (for more details, we refer to Wolfram Alpha).

ALSO READ:

Integration of 2^x

Integration of log(sinx)

Integration of tanx

Integration of secx

FAQs