The integral of root tanx is equal to
∫√tanx dx = $\dfrac{1}{\sqrt{2}}$ tan-1 ($\dfrac{\tan x-1}{\sqrt{2\tan x}}$) + $\dfrac{1}{2\sqrt{2}}$ log $|\dfrac{\tan x+1-\sqrt{2\tan x}}{\tan x+1+\sqrt{2\tan x}}|$ +C
where C denotes an integral constant. In this post, we will learn how to find the integral of square root of tanx.
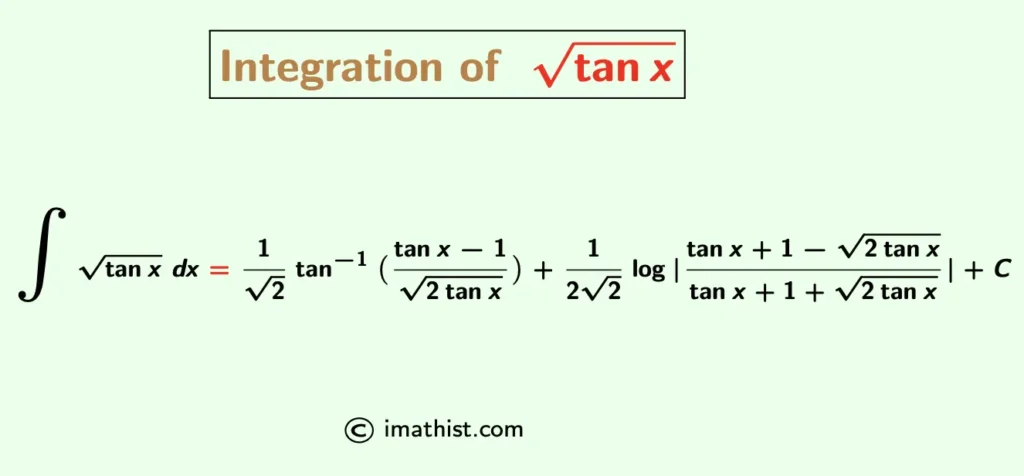
Integration of √tanx
Question: Find ∫√tanx dx.
Solution:
Let tanx=t2.
Differentiating w.r.t x, sec2x dx = 2tdt
∴ dx = $\dfrac{2t dt}{\sec^2x}$ = $\dfrac{2tdt}{1+\tan^2x}$ = $\dfrac{2tdt}{1+t^4}$.
So the given integral of square root of tanx can be written as
∫√tanx dx
= ∫$\dfrac{2t^2 dt}{1+t^4}$
= ∫$\dfrac{(t^2+1)+(t^2-1)}{1+t^4}dt$
= $\int \dfrac{t^2+1}{1+t^4} dt$ + $\int \dfrac{t^2-1}{1+t^4}dt$
Divide both the numerator and the denominator by t2.
= $\int \dfrac{1+\frac{1}{t^2}}{t^2+\frac{1}{t^2}} dt$ + $\int \dfrac{1-\frac{1}{t^2}}{t^2+\frac{1}{t^2}} dt$
= $\int \dfrac{1+\frac{1}{t^2}}{(t-\frac{1}{t})^2+2} dt$ + $\int \dfrac{1-\frac{1}{t^2}}{(t+\frac{1}{t})^2-2} dt$
= I1 + I2 (say).
That is, ∫√tanx dx = I1 + I2 …(∗)
| For I1, put $t-\frac{1}{t}=u$ so that $1+\frac{1}{t^2}=du$. For I2, put $t+\frac{1}{t}=v$ so that $1-\frac{1}{t^2}=dv$. |
Therefore, from (∗) we get that
∫√tanx dx = I1 + I2
= $\int \dfrac{du}{u^2+2} dt$ + $\int \dfrac{dv}{v^2-2} dt$
= $\dfrac{1}{\sqrt{2}}$ tan-1 ($\dfrac{u}{\sqrt{2}}$) + $\dfrac{1}{2\sqrt{2}}$ log $|\dfrac{v-\sqrt{2}}{v+\sqrt{2}}|$ +C
= $\dfrac{1}{\sqrt{2}}$ tan-1 ($\dfrac{t^2-1}{\sqrt{2}t}$) + $\dfrac{1}{2\sqrt{2}}$ log $|\dfrac{t^2+1-\sqrt{2}t}{t^2+1+\sqrt{2}t}|$ +C (putting the above values of u and v)
= $\dfrac{1}{\sqrt{2}}$ tan-1 ($\dfrac{\tan x-1}{\sqrt{2\tan x}}$) + $\dfrac{1}{2\sqrt{2}}$ log $|\dfrac{\tan x+1-\sqrt{2\tan x}}{\tan x+1+\sqrt{2\tan x}}|$ +C.
So the integral of square root of tanx is equal to $\dfrac{1}{\sqrt{2}}$ tan-1 ($\dfrac{\tan x-1}{\sqrt{2\tan x}}$) + $\dfrac{1}{2\sqrt{2}}$ log $|\dfrac{\tan x+1-\sqrt{2\tan x}}{\tan x+1+\sqrt{2\tan x}}|$ +C, where C is an integration constant.
Related Integrals:
FAQs
Q1: What is the integration of root tan x?
Answer: The integration of tan x is equal to ∫√tanx dx = $\dfrac{1}{\sqrt{2}}$ tan-1 ($\dfrac{\tan x-1}{\sqrt{2\tan x}}$) + $\dfrac{1}{2\sqrt{2}}$ log $|\dfrac{\tan x+1-\sqrt{2\tan x}}{\tan x+1+\sqrt{2\tan x}}|$ +C.