Integral of x^2 | How to Integrate x^2? [Solved]

The integral of x^2 (x square) is equal to x³/3+C where C is a constant. The integration formula of x² (x square) is given by

∫x² dx = $\dfrac{x^3}{3}$ +C.

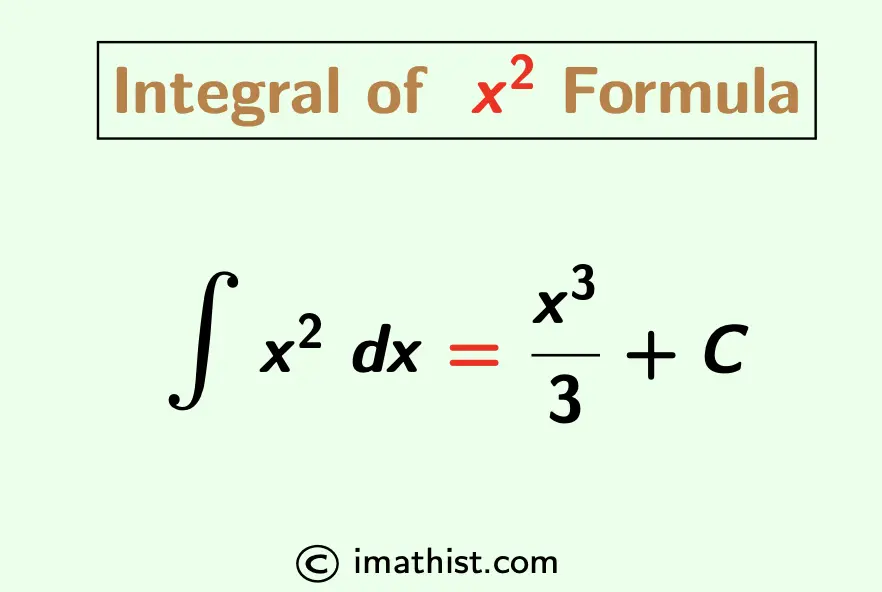
Let us now learn how to integrate x^2 dx.

Integration of x²

Answer: The integral of x square is ∫x² dx = x³/3+C.

Explanation:

To find the integration of x², we will use the power rule of integration formula:

∫xⁿ dx = $\dfrac{x^{n+1}}{n+1}$ +C

with n=2.

So the integration of x^2 will be

∫x² dx = $\dfrac{x^{2+1}}{2+1}$ +C

⇒ ∫x² dx = $\dfrac{x^{3}}{3}$ +C

So the integration of x² is equal to x³/3+C where C is an arbitrary constant, and this is obtained by the power rule of integration.

You Can Read: Integration of xⁿ

What is the integration of tan x?

Integral of secx

Definite Integral of x²

Question: Find the definite integral of x² from 0 to 1, that is, find ∫₀¹ x² dx.

Answer

∫₀¹ x² dx = 1/3.

We have:

∫₀¹ x² dx = [ x³/3 ]₀¹ = 1³/3 – 0³/3 = 1-0 = 1.

So the definite integration of x^2 from 0 to 1 is equal to 1/3.

Read Also: Integration of 1/x

Find Integration of 1/xⁿ

FAQs