Integration of 2^x | 2^x Integration

The integration of 2^x is equal to 2^x/ln2 where ln denotes the natural logarithm, that is, ln=log_e. In this post, we will learn to integrate 2 to the power x with respect to x.

Integration of 2^x Formula

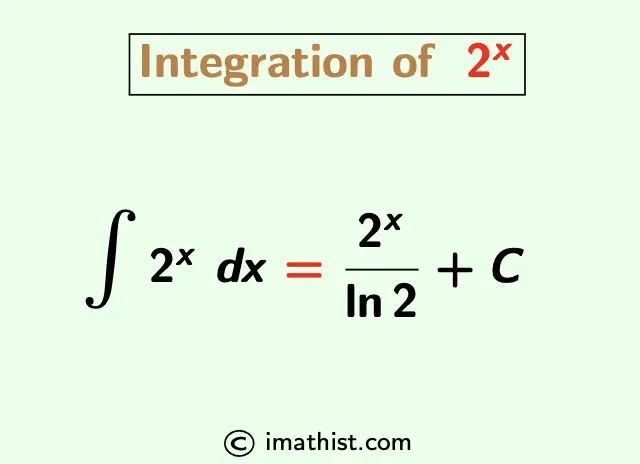
The integration formula of 2^x (2 to the x) with respect to x is given below.

∫ 2^x dx = 2^x/ln2 +C.

How to Integrate 2^x

Now, we will learn how to integrate the function 2 to the x and prove that ∫ 2^x dx = 2^x/ln2 +C.

Proof:

Note that x can be written as x=e^lnx, so we have

2=e^ln2 …(I)

Then, using this identity, the integration of 2^x will be equal to

∫2x dx = ∫(eln2)x dx ⇒ ∫2x dx = ∫exln2 dx …(II)

Let xln2 =z. Then ln2 dx =dz

⇒ dx = dz/ln2

Now, from from (II) we get that

∫2x dx = ∫ez $\dfrac{dz}{\ln 2}$ ⇒ ∫2x dx = $\dfrac{1}{\ln 2}$ ∫ez dz ⇒ ∫2x dx = ez/ln2 as the integration of ez with respect to z is ez. ⇒ ∫2x dx = exln2/ln2 as z=xln2. ⇒ ∫2x dx = 2x/ln2 [Here we have used the identity (I) as exln2 = (eln2)x =(2)x = 2x.]

So the integration of 2^x is equal to 2^x/ln2 where ln2=log_e2. This is obtained by the substitution method of integration and using the exponential identity x=e^lnx.

Video Solution of Integration of 2^x:

Question-Answer

Question: Find the definite integral ∫₀¹ 2^x dx.

Answer:

Using the above integration formula of 2^x, it follows that

∫₀¹ 2^x dx = $\Big[ \dfrac{2^x}{\ln 2}\Big]$

⇒ ∫₀¹ 2^x dx = $\dfrac{2^1}{\ln 2} – \dfrac{2^0}{\ln 2}$

⇒ ∫₀¹ 2^x dx = $\dfrac{1}{\ln 2} (2-1)$

⇒ ∫₀¹ 2^x dx = 1/ln2.

So the definite integral of 2^x from 0 to 1 is equal to ln2.

ALSO READ:

Integration of 1/(1+x²)

Integration of log(sin x)

Integration of $\sqrt{a^2-x^2}$

Integration of $\sqrt{a^2+x^2}$

FAQs