Integration of x^n: Formula, Proof | Integral of x^n

The integration of xⁿ is given as follows: ∫xⁿdx = xⁿ⁺¹/(n+1) + C where C is an integration constant. In this post, we will learn how to find the integral of x to the power n.

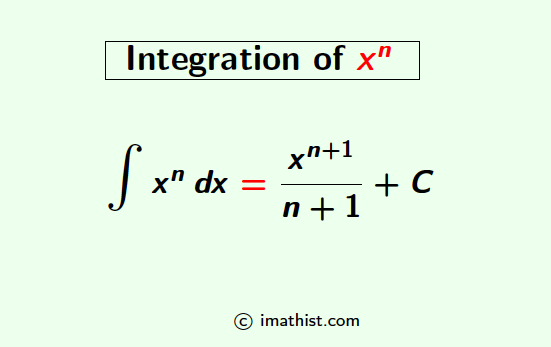
Integration of xⁿ Formula

The integration formula of xⁿ (n-th power x) is given below:

∫xⁿdx = xⁿ⁺¹/(n+1) + C

where C is an integral constant. This rule is called the power rule of integration.

Integration of xⁿ Proof

We know that the derivative of x^k is equal to kx^k-1. Putting k=n+1 we get that

$\dfrac{d}{dx}(x^{n+1})=(n+1)x^n$ …(I)

As the integration (anti-derivative) is the inverse process of derivatives, integrating both sides of (I) we get that

$x^{n+1}=\int (n+1)x^n \ dx +C’$ where C is an integral constant.

⇒ $x^{n+1}=(n+1) \int x^n \ dx +C’$ by the multiplied by constant rule of integration.

⇒ $\dfrac{x^{n+1}}{n+1}= \int x^n \ dx +\dfrac{C’}{n+1}$

⇒ $\int x^n \ dx=\dfrac{x^{n+1}}{n+1}+C$ where $C=-\dfrac{C’}{n+1}$

So the integration of x to the power n is equal to xⁿ⁺¹/(n+1)+C where C is an integration constant.

Few Examples,

1. The integration of x is equal to $\int x \ dx = \dfrac{x^2}{2}+C$
2. The integration of x² (x square) is equal to $\int x^2 \ dx = \dfrac{x^3}{3}+C$
3. The integration of x³ (x cube) is equal to $\int x^3 \ dx = \dfrac{x^4}{4}+C$
4. But, using the above power rule of integration, we cannot find the integration of 1/x. This is because x^-1+1/(-1+1), so we are getting 0 in the denominator. Hence, it does not exist. The integration of 1/x is equal to $\int \dfrac{1}{x} dx = \ln x+C$ where ln denotes the natural logarithm (that is, logarithm with base e).

ALSO READ:

Integration of 1/x

Integration of e^3x

Integration of 1/(1+x²)

Integration of tanx

FAQs