The limit of cosx/x as x approaches 0 does NOT exist. That is, limx→0 (cos x)/x is undefined.
$\lim\limits_{x \to 0} \dfrac{\cos x}{x}$ = NOT exist.
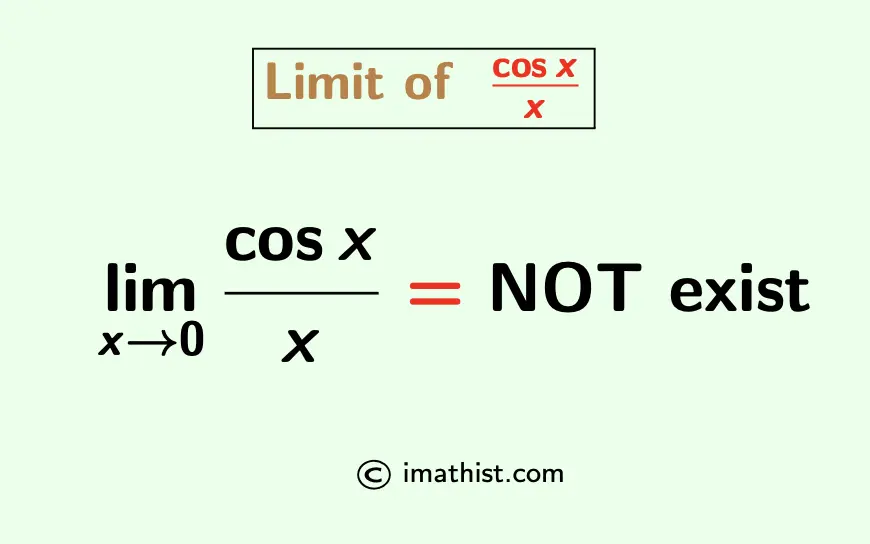
Let us now show that the limit of (cos x)/x when x tends to 0 does not exist.
Limx→0 (cos x)/x
Question: What is the limit of cosx/x when x tends to 0?
Solution:
To show the limit of cosx/x does not exist, we will tend to 0 in two different paths to obtain different limits.
Take xn = 1/n and xn= -1/n.
Both tend to 0 when n→∞.
Observe that $\dfrac{1}{n} \in [0,\frac{\pi}{4}]$, so cos(xn) = cos(1/n) ≥ 1/2.
Therefore,
$\dfrac{\cos x_n}{x_n} \geq \dfrac{1}{2x_n} \geq \dfrac{n}{2}$ …(I)
Similarly, for xn= -1/n, we can show that
$\dfrac{\cos x_n}{x_n} \leq \dfrac{1}{2x_n} \leq \dfrac{-n}{2}$ …(II)
From (I) and (II), we see that $\dfrac{\cos x_n}{x_n}$ approaches to two different limits when xn→0, that is n→∞.
So the limit of cosx/x does not exist. This is proved by taking the limit x→0 in two different paths and obtain two different limits.
Read Also: Limit of cosx/x when x→∞
limx→0 sin(√x)/x | limx→0 sin(x2)/x
limx→0 tanx/x | limx→0(cosx-1)/x
FAQs
Q1: What is the limit of cosx/x when x tends to 0?
Answer: The limit of cosx/x as x tends to 0 does NOT exist.