The limit of sin(x^2)/x as x approaches 0 is equal to 0. The limx -> 0 sin(x2)/x formula is given by
$\lim\limits_{x \to 0} \dfrac{\sin x^2}{x}=0$.
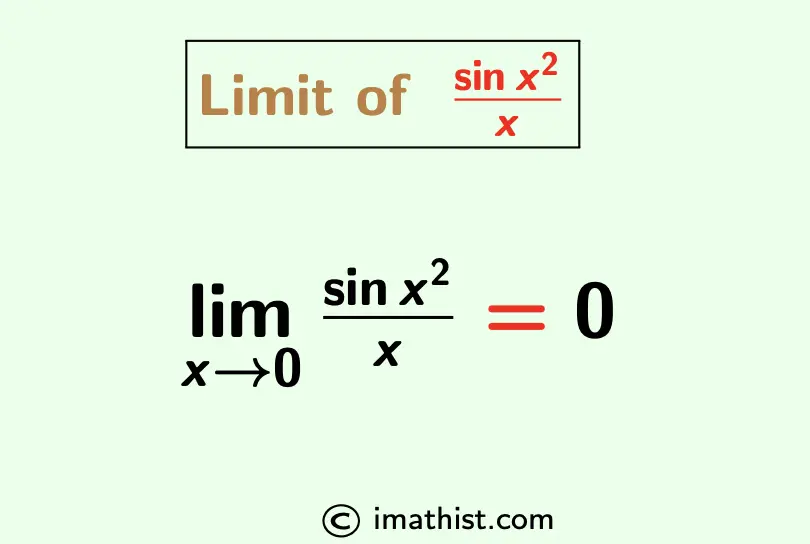
Let us learn how to find the limit of sin x square divided by x when x tends to 0.
Limx → 0 sin(x2)/x
We have
$\lim\limits_{x \to 0} \dfrac{\sin x^2}{x}$
= $\lim\limits_{x \to 0} \dfrac{\sin x^2}{x^2} \times x$
= $\lim\limits_{x \to 0} \dfrac{\sin x^2}{x^2} \times \lim\limits_{x \to 0} x$ by the product rule of limits.
[Let x2=t, so that t→0 as x→0]
= $\lim\limits_{t \to 0} \dfrac{\sin t}{t} \times \lim\limits_{x \to 0} x$
= 1 × 0 using the formula limx→0 sinx/x =1.
= 0
So the limit of sin(x2)/x as x approaches 0 is equal to 0.
More reading: Limit of x/sinx when x→0
Limit of sin(x^2)/2x as x→0
The given limit can be written as follows:
$\lim\limits_{x \to 0} \dfrac{\sin x^2}{2x}$
= $\dfrac{1}{2}\lim\limits_{x \to 0} \dfrac{\sin x^2}{x}$ as 1/2 is a constant.
= $\dfrac{1}{2}\times 0$ by the above limit formula.
= 0.
So the limit of sin(x^2)/2x as x→0 is equal to 0.
Have You Read These Limits? Limit of sin3x/sin2x when x→0
FAQs
Q1: What is the limit of sin(x2)/x when x→0?
Answer: The limit of sin(x2)/x when x→0 is equal to 0, that is, limx→0 sin(x2)/x =0.