Limit of x^1/x as x approaches infinity

The limit of x^1/x as x approaches infinity is equal to 1. This limit is denoted by lim_x→∞ x^1/x, so the formula for the limit of x^1/x when x tends to infinity is given by

lim_x→∞ x^1/x = 1.

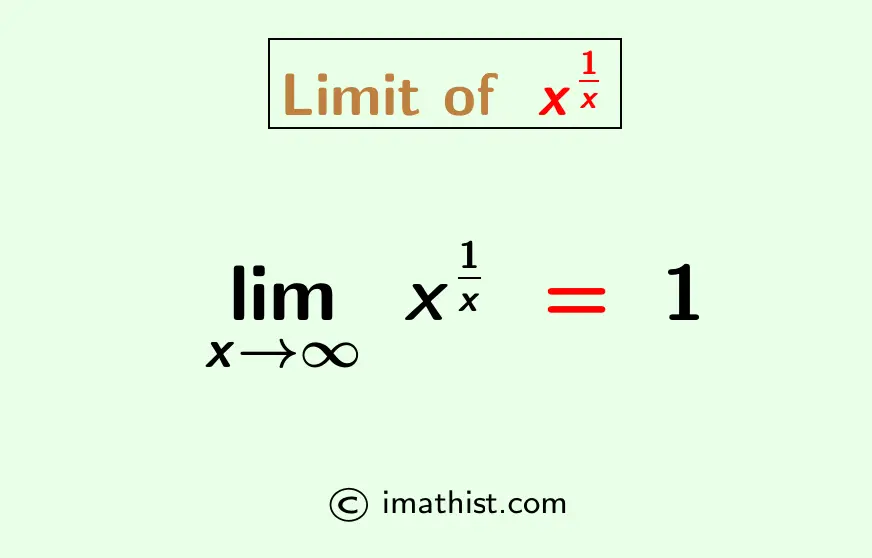
Let us now find the limit of x to the power 1/x when x tends to ∞.

Proof of Limit x^1/x when x→∞

Answer: The limit of x1/x when x→∞ is equal to 1.

Explanation:

Step 1:

Use the exponential formula: $x^y=e^{\ln(x^y)}$. Thus, the given limit will be

lim_x→∞ x^1/x = lim_x→∞ $e^{\ln(x^{1/x})}$

⇒ lim_x→∞ x^1/x = lim_x→∞ $e^{\frac{1}{x} \ln(x)}$ using the formula ln(x^y) = y ln(x).

⇒ lim_x→∞ x^1/x = $e^{\lim\limits_{x \to \infty} \frac{\ln x}{x}}$ …(∗)

Step 2:

Now we compute the limit lim_x→∞ $\dfrac{\ln x}{x}$.

Note that this has the indeterminate form ∞/∞. So using the l’Hospital’s Rule, we obtain that

lim_x→∞ $\dfrac{\ln x}{x}$ = lim_x→∞ $\dfrac{1/x}{1}$ = lim_x→∞ $\dfrac{1}{x}$ = 0.

Step 3:

Using lim_x→∞ ln(x)/x = 0, we deduce from (∗) that

lim_x→∞ x^1/x = e⁰ = 1.

So the limit of x^1/x is equal to 1 when x approaches 0.

More Limits:

lim_x→0 (cosx -1)/x | lim_x→0 cosx/x

lim_x→0 sinx/x | lim_x→0 tanx/x

Limit of (1+ 1/x)^x when x→∞

Limit of cos(1/x) when x→∞

Limit of cos(1/x) when x→0

FAQs