Sec2x Identity | Sec2x Formula

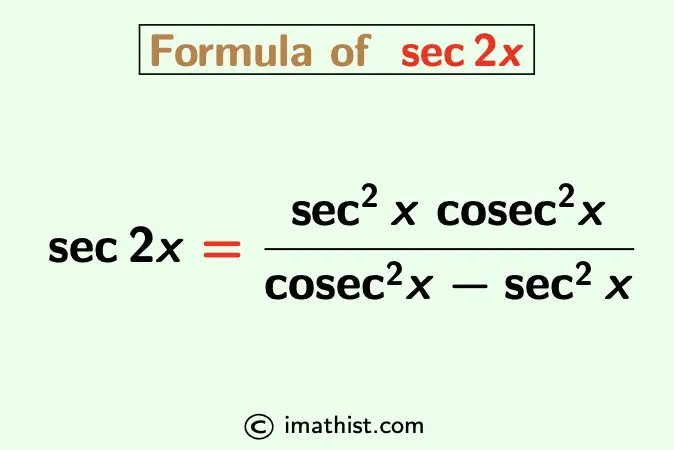
The sec2x identity is given by sec 2x = (sec²x cosec²x) / (cosec²x – sec²x). That is, the formula of sec2x is equal to

sec 2x = $\dfrac{\sec^2x \cdot \text{cosec}^2x}{\text{cosec}^2x – \sec^2x}$

Now, let us learn how to find the sec2x identity

Identity of sec2x

Question: What is the identity of sec2x?

Answer: The sec2x identity is equal to sec2x = (sec²x cosec²x)/(cosec²x – sec²x).

Explanation:

Proof of sec2x Identity: As secx is the reciprocal of cosx, we have that

sec2x = $\dfrac{1}{\cos 2x}$

= $\dfrac{1}{\cos (x+x)}$

= $\dfrac{1}{\cos x \cos x -\sin x \sin x}$ as we know cos(a+b) = cosa cosb -sina sinb

= $\dfrac{1}{\cos^2 x -\sin^2 x}$

= $\dfrac{1}{\frac{1}{\sec^2 x} -\frac{1}{\text{cosec}^2 x}}$, because $\cos x=\dfrac{1}{\sec x}$ and $\sin x =\dfrac{1}{\text{cosec}x}$.

= $\dfrac{1}{\frac{\text{cosec}^2 x -\sec^2 x}{\sec^2 x \cdot \text{cosec}^2 x}}$

= $\dfrac{\sec^2 x \cdot \text{cosec}^2 x}{\text{cosec}^2 x -\sec^2 x}$

So the identity of sec2x is equal to sec2x = (sec²x cosec²x)/(cosec²x – sec²x), and this is the sec2x identity in terms of secx and cosecx.

$\boxed{\sec 2x = \dfrac{\sec^2x \cdot \text{cosec}^2x}{\text{cosec}^2x – \sec^2x}}$

You Can Read: cos3x Formula | sin3x Formula

1-sec2x Formula	cos4x+sin4x Formula
1+cot2x Formula	cos2x-sin2x Formula
cosx cosy Formula	sinx siny Formula

FAQs