Find the Value of sin(3pi/2) | Sin 3π/2 Value

The value of sin(3pi/2), that is, sin 270° is equal to -1. So the formula of sin(3π/2) is given by sin $\frac{3\pi}{2}$ = -1. Here we will learn how to find the value of sin 3pi/2. Note that

• sin(3pi/2) is the value of sine trigonometric function for 3π/2 radians.
• sin(3π/2) = -1.
• sin(3π/2) in degrees is equal to sin 270°.
• sin 270 = -1.

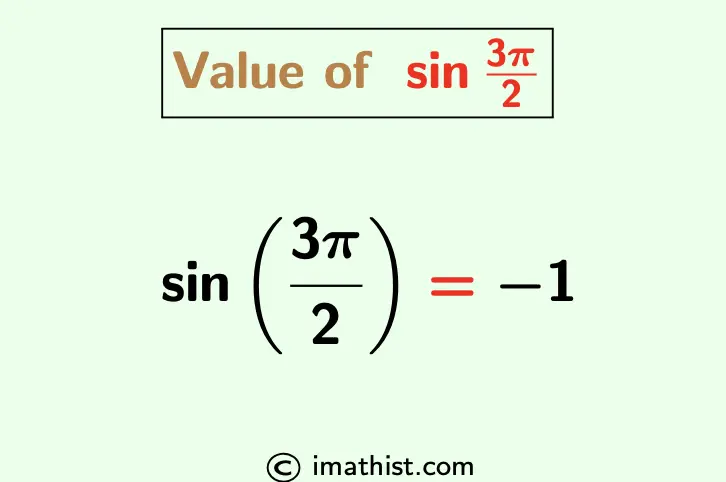
Sin 3pi/2 Value

Question: What is the value of sin(3pi/2)?

Answer: sin(3π/2) is equal to -1.

Explanation:

One can obtain the value of sin(3pi/2) by applying the formula of sin3x in terms of sinx. The formula is given below:

sin3x = 3sinx – 4sin³x.

Put x=π/2. Therefore,

sin(3π/2) = 3sin(π/2) – 4sin³(π/2)

⇒ sin(3π/2) = 3 ⋅ 1 – 4⋅1³ as the value of sin(π/2) is 1.

⇒ sin(3π/2) = 3 – 4

⇒ sin(3π/2) = -1.

So the value of sin 3pi/2 is equal to -1.

Also Read: Sin(π-x) Formula

Formula of sin(a+b) sin(a-b)

Formula of cos(a+b) cos(a-b)

Value of sin(-3pi/2)

By the rule sin(-θ) =-sinθ, we have that

sin(-3π/2) = – sin(3π/2) = -(-1), by the above.

∴sin(-3π/2) = 1.

So the value of sin(-3pi/2) is equal to 1.

More Reading: Value of sin15, cos 15, tan 15

Value of sin75, cos 75, tan 75

FAQs