How to Solve sinx=1/2 [General Solution]

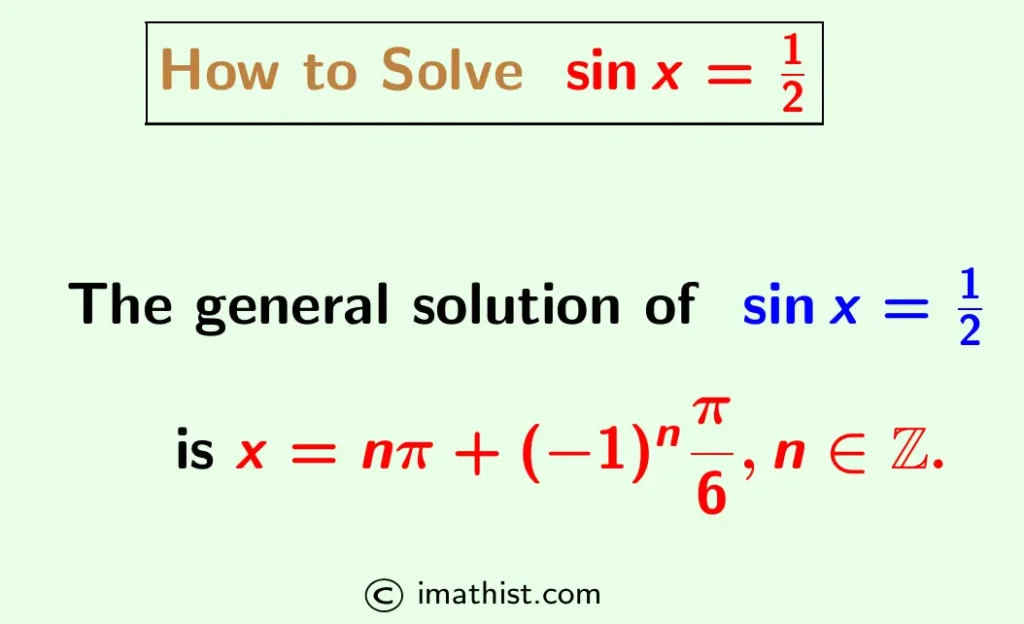
The general solution of sinx=1/2 is x= nπ +(-1)ⁿ π/6 where n ∈ ℤ. In this post, we will learn how to solve sinx = 1/2.

A particular solution of sinx=1/2 is given by x=π/6 as we know that sin(π/6) = 1/2, whereas the general solution of sinx=1/2 is as follows:

$\boxed{x= n \pi + (-1)^n\dfrac{\pi}{6}, n \in \mathbb{Z}}$

General Solution of sinx=1/2

Question: Find the general solution of sinx=1/2.

Answer: The general solution of sinx=1/2 is given by x=nπ+(-1)ⁿπ/6, where n is an integer.

The trigonometric equation sinx = 1/2 can be solved using the following formula.

The general solution of sinx = sinα is given by x=nπ+(-1)nα, where n is an integer.

First note that sin(30°) =1/2.

As 180°=π radian, so we have:

1° = $\dfrac{\pi}{180}$

⇒ 30° = $\dfrac{30 \times \pi}{180}$

⇒ 30° = $\dfrac{\pi}{6}$.

Now,

sinx = 1/2

⇒ sinx = sin(π/6).

Therefore, using the above formula, the general solution of the equation sinx=1/2 is given by x=nπ+(-1)ⁿπ/6, where n is an integer.

Particular Solution: Put n=0. Thus, x = 0⋅π+(-1)0π/6 = π/6 is a particular solution of sinx=1/2.

Question Answer

Q1: What is the general solution of sin2x=1/2?

Answer:

As the general solution of sinx = 1/2 is equal to x=nπ+(-1)ⁿπ/6, n is an integer, it follows that the general solution of sin2x=1/2 is given by

2x=nπ+(-1)ⁿπ/6 ⇒ x = nπ/2 +(-1)ⁿπ/12,

where n is an integer.

Related Articles:

• Solve sinx = 1
• Solve sinx = -1

FAQs