The sum rule of limits says that the limit of the sum of two functions is the same as the sum of the limits of the individual functions. In this post, we will prove the sum/addition rule of limits by the epsilon-delta method.
Question: What is an epsilon-delta proof of the sum law of limits? Here, you will find the answer given below.
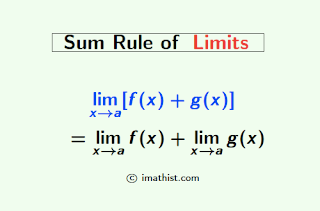
Sum law of Limits
If the limits of two functions f and g exist when x tends to a, then
$\lim_{x \to a}(f+g)$ $=\lim_{x \to a} f + \lim_{x \to a} g$
Proof:
To prove the sum law of limits, we will use the epsilon-delta method. Let $\lim_{x \to a} f(x) = A$ and $\lim_{x \to a} g(x) = B$. So by the epsilon-delta definition of limits, we have the following:
For a given ε>0, there exists δ1>0 and δ2>0 such that
|f(x)-A| < ε/2, whenever 0< |x-a| < δ1 …(I)
and
|g(x)-B| < ε/2, whenever 0< |x-a| < δ2 …(II)
Set δ: = min{δ1, δ2}.
Then whenever 0< |x-a| < δ, we have that
|f(x)+g(x) – (A+B)| = |(f(x) – A) + (g(x) – B)|
≤ |f(x) – A| + |g(x) – B| by the triangle inequality |a+b| ≤ |a|+|b|
≤ ε/2 + ε/2 by (I) and (II)
= ε
Thus, $\lim_{x \to a}(f+g)$ $=\lim_{x \to a} f + \lim_{x \to a} g$ and we have proved the sume rule / addition rule of limits.
Examples
We know that limx→0 x2 =0 and limx→0 1=1.
So by the above sum rule of limits, the limit of x2+1, when x tends to 0, is equal to
limx→0 (x2+1)
= limx→0 x2 + limx→0 1
= 0+1
= 1
FAQs
Q1: What is the sum rule of limits?
Answer: The sum rule of limits is given by limx→0 [f(x)+g(x)] = limx→0 f(x) + limx→0 g(x).