Find the Value of cos(5pi/2) | Cos 5π/2 Value

The value of cos(5pi/2) is equal to 0. The formula of cos(5π/2) is given by

$\boxed{\cos \dfrac{5\pi}{2} = 0}.$

Cos(5pi/2) is the value of cosine trigonometric function for 5π/2 radians. Note that

• cos(5π/2) = 0.
• cos(5π/2) in degrees is equal to cos 450°.
• cos 450° = 0.

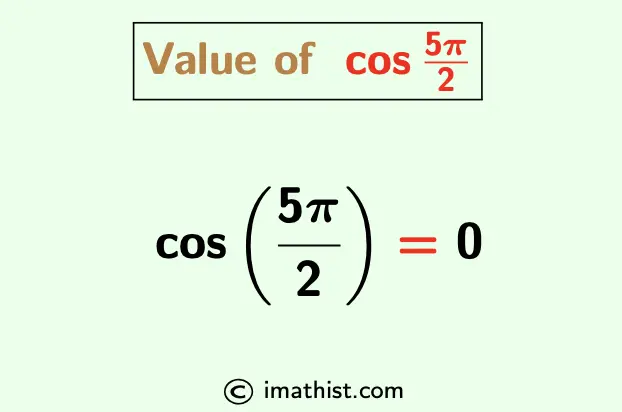
What is the Value of Cos 5pi/2?

Answer: cos(5π/2) is equal to 0.

Explanation:

Note that 5π/2 can be written as follows.

$\dfrac{5\pi}{2}=2\pi +\dfrac{\pi}{2}$.

Now using the formula cos(2π+θ)=cosθ, we obtain that

$\cos(\dfrac{5\pi}{2})=\cos(2\pi +\dfrac{\pi}{2})$.

= $\cos \dfrac{\pi}{2}$

= 0.

So the value of cos 5pi/2 is equal to 0.

Also Read: Sin(π-x) Formula | Value of sin(3π/2)

Value of cos(-5pi/2)

By the rule cos(-θ) = cosθ, we have that

cos(-5π/2) = cos(5π/2) = 0, by the above.

∴ cos(-5π/2) = 0.

Therefore, the value of cos(-5pi/2) is equal to 0.

More Reading:

Formula of sin(a+b) sin(a-b)

Formula of cos(a+b) cos(a-b)

Value of sin15, cos 15, tan 15

Value of sin75, cos 75, tan 75

FAQs